Offset Straight-Tooth Roller Development Using the Discrete Element Method for Applying Granular Mineral Fertilizer
Corresponding email: s.nukeshev@kazatu.kz
Published at : 24 Dec 2024
Volume : IJtech
Vol 15, No 6 (2024)
DOI : https://doi.org/10.14716/ijtech.v15i6.7311
Nukeshev, S., Sugirbay, A., Dulatbay, Y., Tanbaev, K., Yeskhozhin, K., Chen, J., Nazarbayev, Y., Sugirbaeva, Z., 2024. Offset Straight-Tooth Roller Development Using the Discrete Element Method for Applying Granular Mineral Fertilizer. International Journal of Technology. Volume 15(6), pp. 2060-2073
| Sayakhat Nukeshev | Technical Faculty, S. Seifullin Kazakh Agrotechnical University |
| Adilet Sugirbay | - Research School of Veterinary Medicine and Agriculture, Semey State University named after Shakarim - |
| Yerassyl Dulatbay | Research School of Veterinary Medicine and Agriculture, Semey State University named after Shakarim |
| Khozhakeldi Tanbaev | Department of Engineering Technologies and Transport, Sh. Ualikhanov Kokshetau University |
| Kairat Yeskhozhin | Technical Faculty, S. Seifullin Kazakh Agrotechnical University |
| Jun Chen | College of Mechanical and Electronic Engineering, Northwest A?F University |
| Yerassyl Nazarbayev | Technical Faculty, S. Seifullin Kazakh Agrotechnical University |
| Zhanar Sugirbaeva | Department of Higher Mathematics, L.N. Gumilyov Eurasian National University |
This
study aimed to design and evaluate offset straight teeth roller for precise
application of granular mineral fertilizer at low rotation speeds. In the first
stage, the experiment focused on optimizing the gap length between offset
straight-tooth to prevent unintended fertilizer flow and ensure even
distribution. In the second stage, additional gaps on the left and right sides
of offset straight teeth were compared to a single gap. The results of the
comparison showed that adding gaps on the sides improved the discharge rate and
the uniformity of distribution. Therefore, offset straight-tooth roller was
divided into five sections, each with a length of 12 mm. Discrete Element
Method (DEM) simulations showed an actual discharge mass of 148.35 g, producing
a slippage rate of 35.9%. Verification using laboratory equipment with offset
straight-tooth roller showed a discharge of 150 g of mineral granular
fertilizer.
Discrete element method; Interaction properties; Mineral granular fertilizer; Offset straight-tooth roller
The current global tendency is focused on mineral fertilizer applications to meet the precise nutritional needs of plants, maximize yields, and enhance quality. The process is carried out to ensure that crop production contributes to a smooth and healthy food chain, showing the interconnectedness of agriculture, the environment, and human health (Noulas, Torabian, and Qin, 2023). This shows the need to apply granular mineral fertilizer evenly into the soil to promote uniform plant growth, prevent nutrient imbalances, maximize nutrient efficiency, and reduce environmental risks (Bijay-Singh, N., & Tek B. S., 2022; Petrus et al., 2020; Nukeshev, Tanbayev, et al.). In drought conditions, applying a small dose of granular fertilizer has a positive effect, while high dose has no positive effect but negative impact (Nukeshev et al., 2023; Wang et al., 2019; Kaur et al., 2017). Applying high doses of granular fertilizer is associated with a significant positive effect when the soil has enough water during the vegetation period. Therefore, the even application of granular fertilizer is essential, particularly at a high or a small dose.
Metering device is an integral to precision agriculture practices, allowing accurate application of mineral fertilizer based on field variability and crop requirements (Shafi et al., 2019; Stoorvogel, Kooistra, Bouma, 2015). This enables targeted nutrient application, where fertilizer is applied, leading to more efficient resource use and improved crop performance (Ahmad and Dar, 2020). A well-designed metering device ensures that fertilizer is distributed evenly across the entire field. This prevents uneven nutrient distribution that can cause variations in crop growth and yield (Yeskhozhin et al., 2018; Nukeshev et al., 2017; Zhao, Pengfei, et al., 2024; 2016a; 2016b; Zhaksylykova et al., 2016). To achieve even distribution of granular fertilizer, scientists are designing various metering devices. For example, the pin-roller metering device was designed to apply a high dose of granular fertilizer evenly, where the pins of the pin-roller were in the form of a truncated pyramid (Sugirbay et al., 2023; Nukeshev et al., 2019; Sugirbay et al., 2020). The even distribution or coefficient of variation (CV) for granular fertilizer of the pin-roller metering device was 37%. Meanwhile, the CV of 6 and 12 grooved metering devices were 111.13% and 80.74%, respectively. These pin-roller metering devices distribute granular fertilizer evenly, but to provide experiments were printed by 3D printer.
Manufacturing the pin-roller metering device is complex with machine tools. In this context, a fluted-roller metering device has been proven effective for granular fertilizer due to the easy manufacturing process and adjustment of the working length (Bu et al., 2022; Ku?, 2021; Zeng et al., 2020; Ding et al., 2018; Huang et al., 2018; Minfeng et al., 2018; Su et al., 2015; Lv, Yu, and Fu, 2013). However, fluted-roller metering devices discharge unevenly a butch high dose of granular fertilizer, which can be addressed by decreasing the working length and increasing the rotation speed. Increasing the rotation speed of roller does not allow the metering device with a little electric motor, regarding an unmanned aerial vehicle to discharge granular fertilizer on the soil surface (Al-Gaadi et al., 2023; Su et al., 2022).
The recent trend is to drive metering device of the seeders with electric motors to apply variable rates of granular fertilizer. To decrease the CV or increase the even distribution, the grooves of fluted-roller are designed spirally to replace the conventional straight grooved wheel. Although the spiral grooved wheel showed superior uniformity in fertilizer discharge, the complexity of manufacturing increased due to design modifications. A study was conducted using offset spiral teeth fertilizer discharge device (Fang et al., 2024). It was also reported that decreasing the working length to less than 15 mm of spiral grooved metering device led to the discharge of a batch of granular fertilizer (Wang et al., 2023). Another study was conducted using two fluted rollers or gap squeeze gear-type discharger to dispense granular fertilizer. This was based on the principle of half-cycle superposition of fertilizer discharge curve (Dun et al., 2023a; 2023 b; 2021). Fertilizer apparatus featuring arc gears operates on the principle of releasing fertilizer through clearance within the circular arc discharging gear. This gear maintains a consistent gap, while the teeth spaced move continuously and alternately. The design enhances the consistency of fertilizer discharge flow, preventing common issues such as blockages and intermittent discharge found in conventional fertilizer dispensers. However, increasing the gap between the gears to apply a high dose of granular fertilizer at low rotation speeds is not recommended because of free flow. The use of two rollers also complicates the design of the metering device.
Based on the description, this study aimed to propose a new design featuring a six-grooved roller for discharging high doses. The design was similar to offset spiral teeth fertilizer discharge device but with straight-teeth and a gap between offset. The objective was to effectively use the space between the teeth for evenly discharging high dose of granular fertilizer. To improve the even distribution of granular fertilizer, the six-grooved roller, with a length of 60 mm, was divided into identical left and right parts, offset axially by 30 degrees, with the gap located on the middle side. The first task was to determine the influence of gap located in the middle with various parameters, which was optimized to prevent the free flow of granular fertilizer. The second task was to add more gaps on the left and right sides of roller and compare the results. The discharged granular fertilizer mass, slippage rate (SR), and CV in the discharged mass were used as parameters to compare the influence of different settings.
2.1. The design and parameters of offset straight-tooth six-grooved roller with the gap
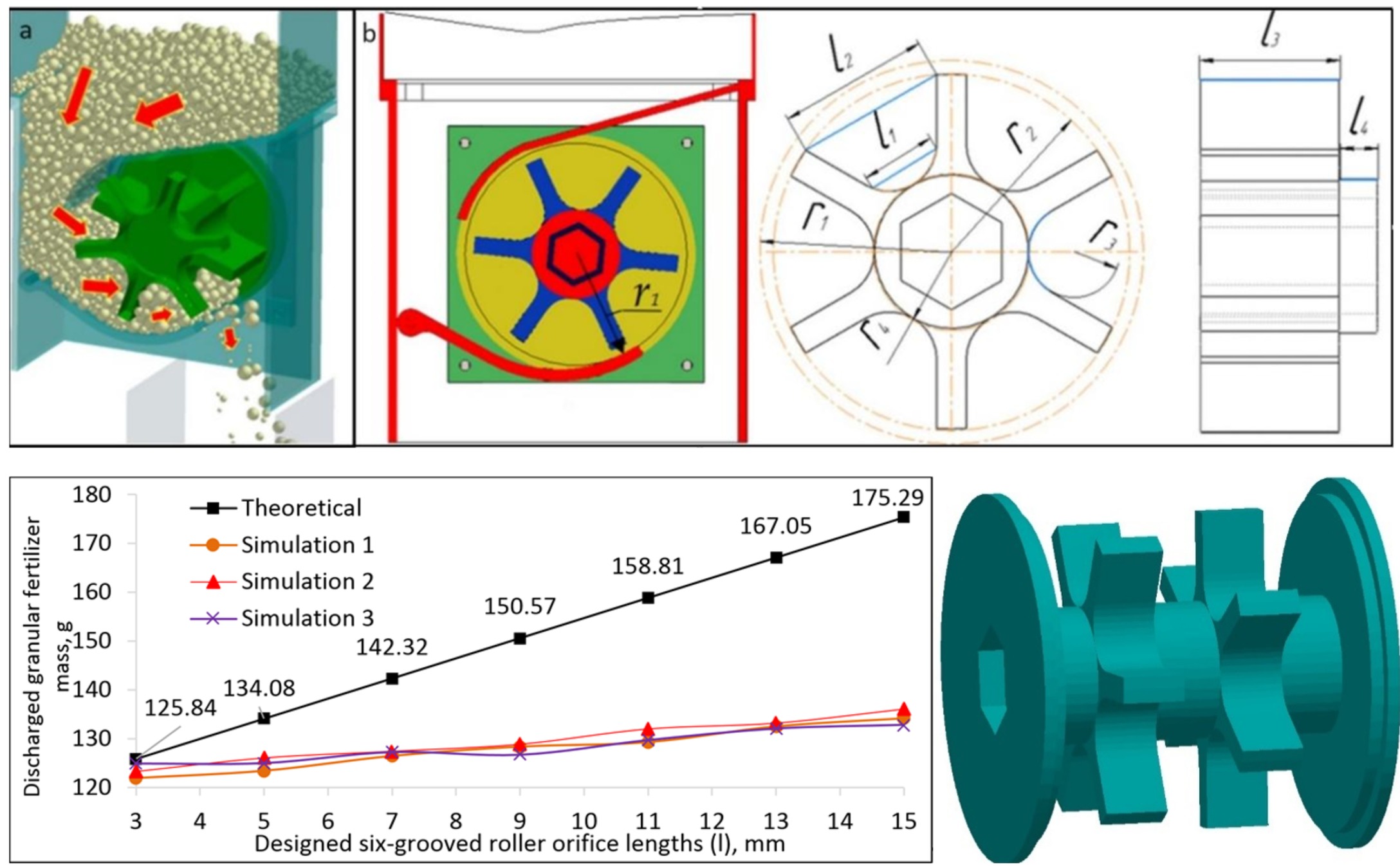
The laboratory equipment required for conducting the experiment consists of several components. These include a tank for storing granular fertilizer (1), a casing for the metering device (2), a specially designed six-grooved roller (3), left and right side caps (4), a bottom cap (5), and left and right side discs (6) and bolts (7, 8) (Figure 1a). The casing of the metering device is connected to the tank using bolts and the orifice at the bottom of the tank is in line with the open area of the casing on the top. To direct granular fertilizer, there is a plate inclined at 20 degrees, and the end of the plate is curved along the orifice of the casing (Figure 1b). The middle side view of the metering device casing showed the radius of the casing orifice is 30.25 mm. The metering device casing contained orifices to fix the position of the left and right side caps (4) and the bottom cap (5). The parameters of the disks and caps are showed in Figures 1c and 1d. The inner radius of the disc is fitted into the orifice of the metering device casing, while the outer radius of the disc is connected to the casing. The left and right caps are secured to the metering device casing with bolts, ensuring the positioning of the discs relative to the metering device casing. The parameters of the designed six-grooved roller are shown in Figure 1e.
Figure 1 Design and parameters of the of the metering device: a. View of the metering device: 1. Granular fertilizer tank; 2. Metering device casing; 3. Designed six-grooved roller; 4. Right side cap; 5. Bottom cap; 6. Right side disc; 7. Bolt; 8. Bottom cap bolt; b. Metering device case parameters; c. Disc parameters; d. Left and right side cap parameters; e. Designed six-grooved roller parameters; f. Bottom cap parameters.
The designed six-grooved roller is axially in line with the discs and metering device orifice, which can freely rotate in both clockwise and counterclockwise directions. The left and right discs are connected to the six-grooved roller and rotate together. The six-grooved roller collar contains identical left and right parts offset axially by 30 degrees. Moreover, there is a gap located on the middle side of the six-grooved roller, which is essential for determining the optimum length (l) of the gap. The bottom cap is shown in Figure 1f, which is fixed to the metering device casing on one side with a cotter pin and on the other side with a bolt.
2.2. The theoretical calculation of the designed six-grooved metering device working volume
The working volume of the designed six-grooved roller per one revolution is calculated by summing the free space of the six-grooved roller and determining the volume of the active layer (equation 1). The active layer volume is considered because of the distance between the six-grooved roller and bottom cap due to the interaction between granular fertilizer. Figure 2 shows granular fertilizer flow inside the metering device and the main parameters for calculating the working volume of the designed six-grooved roller. Granular fertilizer by their gravity move from the tank and fill the free space of the six-grooved roller.
Figure 2 Designed six-grooved metering device: a. The flow of granular fertilizer b.
The main parameters to calculate the working volume of the designed six-grooved roller are showed in Equation 1:
where, Vwv – the working volume of the six-grooved roller; Vr – the free space of the six-grooved roller; Val – the active layer volume of the six-grooved roller.
The volume of the six-groover roller free space is determined as (Equation 2):
where, Vg- the volume of the single groove; Vo-the volume of the gap in the middle of roller.
The volume of the single groove is determined as (Figure 3) (Equation 3, 4):
where, S1- upper segment area; S2-bottom segment area; S3-middle area in the shape of trapezoid.
where, 1-angle of the groove, 60 degrees; -angle of the chord, 120 degrees;
- radius of roller, 30 mm; l1-the length of the bottom chord, 13.86 mm; l2- the length of the upper chord, 30 mm; l3-the length of the groove, mm; h - the distance between the upper and bottom chords, 13.98 mm.
Figure 3 The parameters to calculate the volume of the single groove free space.
The volume of the gap is determined as: (Equation 5)
where, r4-the distance till the groove bottom; l4-the length of the gap.
The volume of the active layer is determined as (Equation 6):
where, r1-the distance to the bottom cap, 35 mm.
The overall working volume of the designed six-grooved metering device is determined as (Equation 7):
Granular fertilizer weight per one revolution of the six-grooved roller is determined as (Equation 8):
where, -granular fertilizer bulk density, 946.4 kg m-3.
In this study l4 is the main parameter to optimize, which influences granular fertilizer weight per one revolution (l=2l4), The length of the groove (l3) depends on (l4) (l3+l4=30). When the length of the gap is more than optimal, granular fertilizer particles moves on the surface of the bottom cap and fall free. This condition can be prevented by decreasing the free flow of granular fertilizer gap length (l4). However, a decrease in the gap length may reduce granular fertilizer weight per one revolution. The hypothesis of this study was to determine the optimum length of the designed six-grooved roller gap to increase granular fertilizer flow and improve the even distribution.
2.3. Discrete element method (DEM) model and interaction properties
The simulation was conducted using the DEM model (Siripath, Suranuntchai, and Sucharitpwatskul, 2024). Material properties for the Hertz-Mindlin no-slip numerical model were obtained from existing literature. However, the density of granular fertilizer was determined through experimental data. The shear modulus and Poisson's ratio values for granular fertilizer correlated with the experimental results. The particle size distribution played a significant role in the interaction properties between particles and materials, thereby affecting the overall experimental results. Boundary conditions were not defined, as the primary focus was on particle behavior within the domain. The Euler method was used for time integration in the Hertz-Mindlin no-slip model, with a Rayleigh time-step set at 30%. The simulation grid had an estimated cell radius of 3 mm. Table 2 shows the values for interparticle and particle-material interactions.
Table 1 Material mechanical properties
Table 2 Values for interparticle and particle-material interaction properties (Sugirbay et al., 2021)
2.4. Designed six-grooved offset straight tooth metering device simulation with various parameters on DEM
The simulation process of the designed six-grooved metering device depending on time is shown in Figure 4. The metering device geometry was inserted into the DEM and the interaction properties were set based on previous parameters. The factory was generated to create granular fertilizer on top of the tank (black one). The box was generated to gather the discharged granular fertilizer under the metering device casing (red one). The top of the box was open.
Initially, the factory started generating 5000 granular fertilizer particles per second. After one second, the six-grooved roller was filled and started rotating counterclockwise on its axis at a speed of 10 rpm. At 2 seconds, some granular fertilizer particles were discharged and had fallen into the box. At 2.3 seconds, 10,000 granular fertilizer particles finished gathering into the tank. It was considered that collecting the discharged granular fertilizer particles during the 8 seconds starting from 2 seconds until 10 seconds would be satisfactory to compare the six-grooved roller with various parameters. The mass flow sensor (MFS), shown by cylindrical green grid on the last figure on DEM, measures the dynamic granular fertilizer mass and exports the data into an Excel file for analysis.
Figure 4 Simulation process of the designed six-grooved metering device on DEM.
2.4.1. Measuring the discharged granular fertilizer mass and particle slippage on DEM per revolution
The triplicated simulation of the designed six-grooved roller with various gap lengths (l) ranging from 3 mm to 15 mm, in increments of 2 mm, was conducted for seven parameters. The exported data was analyzed using an Excel file and the discharged granular fertilizer mass per revolution of roller was calculated. With roller rotation speed of 10 rpm, data from 2 to 8 seconds was measured to calculate the discharged granular fertilizer mass per revolution of roller. The theoretical calculation of the discharged granular mass (Qwv) was compared with the simulation results on DEM (Qsim). The comparison was required to determine the slippage between the discharged granular fertilizer particles based on the designed six-grooved roller with various gap lengths (l). The SR is determined as follows (Equation 9):
2.4.2. Calculation of the CV on DEM
The data exported to the Excel file was analyzed to calculate the CV of the discharged granular mass. This was performed by conducting triplicated simulations of the designed six-grooved roller with various gap lengths (l) ranging from 3 mm to 15 mm, in increments of 2 mm, for seven parameters. Based on each simulation, 198 data points were collected during the 8 seconds from 2 to 10 seconds, with a time interval of 0.04 seconds between each data point. The final data point was subtracted from the previous data point to determine the discharged granular fertilizer mass over 0.04 seconds, leading to 197 data points. To calculate the CV, the standard deviation of these 197 data points was divided by the mean and multiplied by 100.
2.5. Laboratory equipment to verify simulation results
To verify the simulation results, laboratory equipment was constructed, as shown in Figure 5. The equipment included granular fertilizer tank (1), a metering device with offset straight-tooth roller (2), a DC electric motor with a gearbox (CHR-GM37-3429-ABHL) (3), an IRF520 driver (4), an ESP32 DEVKIT V1 microcontroller (5), a laptop (6), and a container (7). Granular fertilizer tank was filled with fertilizer and connected to the metering device with offset straight-teeth roller. The parameters of roller were expected to be validated by the simulation results. The metering device was driven by a DC electric motor powered by a 12V battery through the IRF520 driver. The gearbox had a reduction ratio of 1:450, while the IRF520 driver was controlled by the ESP32 DEVKIT V1 microcontroller. The DC electric motor also included a rotary encoder for PID closed-loop speed control. The rotation speed of the metering device with offset straight-tooth roller was 10 rpm. Subsequently, the discharged granular fertilizer was collected in the container, and the mass was measured with a precision of 0.01 g. The laboratory experiment was conducted five times and the mean mass of the discharged granular fertilizer was determined.
3.1. The results of the theoretical analysis and simulation on DEM with the gap in the middle
In the first task, designed six-grooved roller with various gap lengths (l) is investigated. The results of the theoretical analysis and three replications of the simulation are shown in Figure 6. The theoretical discharged granular fertilizer mass increased significantly from 125.84 g to 175.29 g, as the designed six-grooved roller gap length rose from 3 mm to 15 mm. However, the simulated discharged granular fertilizer mass increased only slightly when the designed six-grooved roller gap length rose from 3 mm to 15 mm. The mean of three replications of the simulation on DEM is showed in Figure 7. The regression coefficient of the linear trend line of the mean of the three simulations on DEM was found to be 0.9926. This showed a linear increase in the discharged granular fertilizer mass simulated on DEM. The simulated discharged granular fertilizer mass mean increased slightly from 123.35 g to 134.30 g, as the designed six-grooved roller gap length rose from 3 mm to 15 mm. The SR increased from 2.02% to 30.52%, along with the increase in gap length. This shows that increasing the designed six-grooved roller gap length from 3 mm to 15 mm leads to more inefficient use of working due to particle slippage. Therefore, there are particle-particle and particle-material interactions. When roller rotates, the material moves the particles which further adjusts the adjacent particles. As the gap length of the designed six-grooved roller increases, the influence of the material on the particles decreases, causing a rise in particle slippage.
Due to particle slippage, increasing the designed six-grooved roller gap length from 3 mm to 15 mm caused a mass increase of only 10.95 g for the discharged granular fertilizer. The direction of discharged granular fertilizer simulated using DEM is shown in Figure 7. The velocity of the particles increases from the center of roller to bottom cap, changing color from gray to black accordingly (Figure 8a). A hypothesis regarding particle slippage is shown in Figure 8 b. Granular fertilizer particles inside the groove move towards the middle as the particles leave the bottom cup due to bulk angle of repose (Bu et al., 2022), creating free space. After filling the free space, fewer granular fertilizer particles move from the tank to the designed six-grooved roller gap.
The increase in gap length significantly improves the even distribution of granular fertilizer, with the CV decreasing from 63.18% to 38.73% (Figure 9). The trend line of the CV is linearly dependent on the designed six-grooved roller gap length, with a regression coefficient of 0.9626 for the linear trend line of the mean CV across three DEM simulations. Moreover, the designed six-grooved roller gap length should not exceed 15 mm. This is to ensure free flow of granular fertilizer particles from the metering device when the six-grooved roller is not rotating and the seeder is moving in the transport position.
Figure 6 Comparison of theoretical and simulation results of three replications using DEM.
Figure 7 The mean mass of discharged granular fertilizer from three replications simulated using DEM.
Figure 8 The direction of discharged granular fertilizer simulated using DEM: a. Left side view; b. Bottomview.
Figure 9 The mean CV of discharged granular fertilizer flow from multiple replications simulated using DEM.
3.2. The influence of the additional gaps on the left and right sides
Additional gaps were added on the left and right sides of roller after determining gap influence in the second task. The comparison of the grooved roller with a gap in the middle, left, and right sides is shown in Figures 10a and 10b. In this case, roller length was divided into five parts to ensure symmetric section of left and right. Therefore, the length of each gap was 12 mm. The theoretical discharged granular fertilizer mass of the grooved roller with gaps in the middle, left, and right sides was calculated according to the equation shown in Figure 6, leading to 201.61 g. The simulation results using DEM showed that the discharged granular fertilizer mass was 148.35 grams and the SR was 35.9%, with CV of 30.94%.
Figure 10 The position of roller gap: a. In the middle; b. In the middle, left, and right sides.
The advantage of offset straight-teeth roller is universal, allowing measurement of granular and cylindrical granulated organic fertilizer with bigger sizes. Organic fertilizer significantly increases the drought resistance index of barley and millet compared to mineral fertilizer in both current and warmed climate conditions. Verification using laboratory equipment with offset straight-tooth roller confirmed a discharge of 150 g of mineral granular fertilizer.
In conclusion, this study aimed to design and evaluate offset straight-teeth roller for precise granular mineral fertilizer application. The innovative roller design was tested in different configurations, namely a single gap in the middle with additional gaps on the left and right sides. The addition of side gaps significantly improved the uniformity and mass of the discharged fertilizer. The theoretical discharge mass was calculated to be 201.61 g, while the actual mass measured through DEM simulation was 148.35 g, producing a SR of 35.9%. The results showed that increasing the gap length led to a rise in particle slippage. This caused an improvement in the even distribution of fertilizer, as shown by a decrease in the CV from 63.18% to 38.73%. Verification using laboratory equipment with offset straight-tooth roller confirmed a discharge of 150 g of mineral granular fertilizer. The results showed the importance of optimizing gap parameters to balance discharge efficiency and uniformity. This suggested that the proposed grooved roller design could enhance the precision of fertilizer application, contributing to more sustainable and efficient agricultural practices.
This study was funded by the Ministry of Science and Higher Education of the Republic of Kazakhstan, grant number AP19674514.
Ahmad, S.F., Dar, A.H., 2020. Precision Farming for Resource Use Efficiency. In: Resources use Efficiency in Agriculture, pp. 109–135 https://link.springer.com/chapter/10.1007/978-981-15-6953-1_4 Al-Gaadi, K.A., Tola, E., Alameen, A.A., Madugundu, R., Marey, S.A., Zeyada, A.M., Edrris, M.K., 2023. Control and Monitoring Systems Used in Variable Rate Application of Solid Fertilizers: A Review. Journal of King Saud University - Science, Volume 35(3), p. 102574. https://doi.org/10.1016/j.jksus.2023.102574 Bijay-Singh, N., and Tek B. S., 2022. The Effects of Adequate and Excessive Application of Mineral Fertilizers on the Soil. Elsevier eBooks, 2022, pp. 369–381. https://doi.org/10.1016/B978-0-12-822974-3.00051-3 Bu, H., Yu, S., Dong, W., Zhang, L., Xia, Y., 2022. Analysis of the Effect of Bivariate Fertilizer Discharger Control Sequence on Fertilizer Discharge Performance. Agriculture, Volume 12, p. 1927. https://doi.org/10.3390/agriculture12111927 Ding, S., Bai, L., Yao, Y., Yue, B., Fu, Z., Zheng, Z., Huang, Y., 2018. Discrete Element Modelling (DEM) of Fertilizer Dual-Banding with Adjustable Rates. Computers and Electronics in Agriculture, Volume 152, pp. 32–39. https://doi.org/10.1016/j.compag.2018.06.044 Dun, G., Gao, Z., Liu, Y., Ji, W., Mao, N., Wu, X., Liu, W., 2021. Optimization Design of Fertilizer Apparatus Owned Arc Gears Based on Discrete Element Method. International Journal of Agricultural and Biological Engineering, Volume 14, pp. 97–105. 10.25165/j.ijabe.20211402.5719 Dun, G., Mao, N., Ji, X., Zhang, F., 2023a. Simulation Analysis and Test of Gap Squeeze Gear Type Fertilizer Discharger for Granular Fertilizer Containing Caking. Available Online at: https://doi.org/10.21203/rs.3.rs-2855025/v1, Accessed on 08 20, 2024 Dun, G., Wu, X., Ji, X., Liu, W., Mao, N., 2023b. Optimization Design and Experiment for Precise Control Double Arc Groove Screw Fertilizer Discharger. Agriculture, Volume 13, p. 1866. https://doi.org/10.3390/agriculture13101866 Fang, L., Yang, W., Luo, X., Guo, H., Song, S., Liu, Q., Xie, H., Chen, W., Lu, J., Peng, Z., Li, G., 2024. Development and Optimization of an Offset Spiral Tooth Fertilizer Discharge Device. Agriculture, Volume 14, p. 329. https://doi.org/10.3390/agriculture14020329 Huang, Y., Wang, B., Yao, Y., Ding, S., Zhang, J., Zhu, R., 2018. Parameter Optimization of Fluted-Roller Meter Using Discrete Element Method. International Journal of Agricultural and Biological Engineering, Volume 11(6), pp. 65–72. https://www.ijabe.org/index.php/ijabe/article/view/3573 Kaur, G., Zurweller, B., Nelson, K., Motavalli, P., Dudenhoeffer, C., 2017. Soil Waterlogging and N Fertilizer Management Effects on Corn and Soybean Yields. Agronomy Journal, Volume 109, pp. 97–106. https://doi.org/10.2134/agronj2016.07.0411 Ku?, E., 2021. Seed Damage Test for Roller-Type Device Designed at Different Flute Helical Angles. International Journal of Agriculture and Wildlife Science, Volume 7(3), pp. 495–502. https://doi.org/10.24180/ijaws.938870 Lv, H., Yu, J., Fu, H., 2013. Simulation of The Operation of a Fertilizer Spreader Based on an Outer Groove Wheel using a Discrete Element Method. Mathematical and Computer Modelling, Volume 58(3), pp. 842–851. https://doi.org/10.1016/j.mcm.2012.12.017 Minfeng, J., Yongqian, D., Hongfeng, Y., Haitao, L., Yizhuo, J., Xiuqing, F., 2018. Optimal Structure Design and Performance Tests of Seed Metering Device with Fluted Rollers for Precision Wheat Seeding Machine. IFAC-PapersOnLine, Volume 51(17), pp. 509–514. https://doi.org/10.1016/j.ifacol.2018.08.158 Noulas, C., Torabian, S., Qin, R., 2023. Crop Nutrient Requirements and Advanced Fertilizer Management Strategies. Agronomy, Volume 13(8), p. 13082017. https://doi.org/10.3390/agronomy13082017 Nukeshev, S., Eskhozhin, D., Lichman, G., Karaivanov, D., Zolotukhin, E., Syzdykov, D., 2016a. Theoretical Substantiation of The Design of a Seeding Device for Differentiated Intra Soil Application of Mineral Fertilizers. Acta Universitatis Agriculturae et Silviculturae Mendelianae Brunensis, Volume 64(1), pp. 115–122. 10.11118/actaun201664010115 Nukeshev, S., Eskhozhin, K., Eskhozhin, D., Syzdykov, D., 2017a. Justification of Design and Parameters of Seeding Unit for Fertilizers. Journal of the Brazilian Society of Mechanical Sciences and Engineering, Volume 39(4), pp. 1139–1149. 10.1007/S40430-016-0588-5 Nukeshev, S., Slavov, ?., Karaivanov, D., Balabekova, A., Zhaksylykova, Z., 2019. Forced Vibrations of the Hopper of Fertilizer Applying Machine. Mechanics, Volume 24(6), pp. 798–804. https://doi.org/10.5755/j01.mech.24.6.22464 Nukeshev, S., Tanbayev, K., Ramaniuk, M., Kakabayev, N., Sugirbay, A., & Moldazhanov, A., 2024. Spray Angle and Uniformity of the Flat Fan Nozzle of Deep Loosener Fertilizer for Intra-Soil Application of Fertilizers. AgriEngineering, 6(2), 1365-1394. https://doi.org/10.3390/agriengineering6020079 Nukeshev, S., Yeskhozhin, K., Karaivanov, D., Ramaniuk, M., Akhmetov, E., Saktaganov, B., Tanbayev, K., 2023. A Chisel Fertilizer for In-Soil Tree-Layer Differential Application in Precision Farming. International Journal of Technology, Volume 14(1), pp. 109–118. https://doi.org/10.14716/ijtech.v14i1.5143 Nukeshev, S., Yeskhozhin, K., Tokusheva, M., Zhazykbayeva, Z., 2016b. Substantiation of the Parameters of the Central Distributor for Mineral Fertilizers. International Journal of Environmental and Science Education, Volume 11, pp. 7932–7945. https://files.eric.ed.gov/fulltext/EJ1117306.pdf Petrus, H.T.B.M., Putera, A.D.P., Wangi, I.P., Ramadhian, M.A., Setiawan, H., Prasetya, A., 2020. Characterization of Nitrogen Release in Modified Controlled-Release-Fertilizer using Rice Husk Biochar. International Journal of Technology, Volume 11(4), pp. 774–783. https://doi.org/10.14716/ijtech.v11i4.3520 Shafi, U., Mumtaz, R., García-Nieto, J., Hassan, S.A., Zaidi, S.A.R., Iqbal, N., 2019. Precision Agriculture Techniques and Practices: From Considerations to Applications. Sensors, Volume 19(17), p. 3796. https://doi.org/10.3390/s19173796 Siripath, N., Suranuntchai, S., Sucharitpwatskul, S., 2024. Modeling Dynamic Recrystallization Kinetics in BS 080M46 Medium Carbon Steel: Experimental Verification and Finite Element Simulation. International Journal of Technology, Volume 15(5), pp. 1292–1307. https://doi.org/10.14716/ijtech.v15i5.6770 Stoorvogel, J.J., Kooistra, L., Bouma, J., 2015. Managing Soil Variability at Different Spatial Scales as a Basis for Precision Agriculture. In: Soil-Specific Farming: Precision Agriculture, Lal, R., Stewart, B.A., (ed.), pp. 37–72. https://doi.org/10.1201/b18759-6 Su, D., Yao, W., Yu F., Liu, Y., Zheng, Z., Wang, Y., Xu, T., Chen, C., 2022. Single-Neuron PID UAV Variable Fertilizer Application Control System Based on a Weighted Coefficient Learning Correction. Agriculture, Volume 12, p. 1019. https://doi.org/10.3390/agriculture12071019 Su, N., Xu, T., Song, L.T., Wang, R., Wei, Y.Y., 2015. Variable Rate Fertilization System with Adjustable Active Feed-Roll Length. International Journal of Agricultural and Biological Engineering, Volume 8, pp. 19–26. 10.3965/j.ijabe.20150804.1644 Sugirbay, A., Zhao, J., Nukeshev, S., Chen, J., Nikolay, Z., Bu, L., Sugirbayeva, Z., Hu, G., Marat, M., Wang, Z., 2021. Calibration Strategy to Determine the Interaction Properties of Fertilizer Particles Using Two Laboratory Tests and DEM. Agriculture, Volume 11(7), p. 592. https://doi.org/10.3390/agriculture11070592 Sugirbay, A., Zhao, K., Liu, G., Nukeshev, S., Chen, J., Hu, G., Bu, L., Chen, Y., Jin, H., Zhang, S., Nikolay, Z., Marat, M. 2023. Investigation of the Pin-Roller Metering Device and Tube Effect for Wheat Seeds and Granular Fertilizers Based on DEM. International Journal of Agricultural and Biological Engineering, Volume 16(2), pp. 103–114. https://10.25165/j.ijabe.20231602.7721 Sugirbay, A.M., Zhao, J., Nukeshev, S.O., Chen, J., 2020. Determination of Pin-Roller Parameters and Evaluation of The Uniformity of Granular Fertilizer Application Metering Devices in Precision Farming. Computers and Electronics in Agriculture, Volume 179, p. 105835. https://doi.org/10.1016/j.compag.2020.105835 Wang, J., Wang, R., Ju, J., Song, Y., Fu, Z., Lin, T., Chen, G., Jiang, R., Wang, Z., 2023. Study on the Influence of Grooved-Wheel Working Parameters on Fertilizer Emission Performance and Parameter Optimization. Agronomy, Volume 13, p. 2779. https://doi.org/10.3390/agronomy13112779 Wang, Y., Zhang, X., Chen, J., Chen, A., Wang, L., Guo, X., Gao, Q., 2019. Reducing Basal Nitrogen Rate to Improve Maize Seedling Growth, Water and Nitrogen Use Efficiencies Under Drought Stress by Optimizing Root Morphology and Distribution. Agricultural Water Management, Volume 212, pp. 328–337. https://doi.org/10.1007/s11104-024-06779-4 Yeskhozhin, D.Z., Nukeshev, S.O., Lichman, G.I., Akhmetov, Y.S., Yeskhozhin, K.D., 2018. The Lobe-Type Agitator Parameters of the Seed-Metering Unit in the Seed-Fertilizer Drill Justification. Agricultural Machinery and Technologies, Volume 12(5), pp. 20–24. https://doi.org/10.22314/2073-7599-2018-12-5-20-24 Zeng, S., Tan, Y., Wang, Y., Luo, X., Yao, L., Huang, D., Zewen, M., 2020. Structural Design and Parameter Determination for Fluted-Roller Fertilizer Applicator. International Journal of Agricultural and Biological Engineering, Volume 13, pp. 101–110. 10.25165/j.ijabe.20201302.4999 Zhaksylykova, Z., Eskozhin, D., Nukeshev, S., Akhmetov, Y., Yeskhozhin, K., 2016. Design of The Construction and Parameters Justification of Stud-Belt Seeding Machine for Application of The Main Dose of Mineral Fertilizer. International Journal of Environmental and Science Education, Volume 11, pp. 12959–12972. http://www.ijese.net/makale/1773.html Zhao, Pengfei, et al., 2024. Investigation of Seeding Performance of a Novel High-speed Precision Seed Metering Device Based on Numerical Simulation and High-speed Camera. Computers and Electronics in Agriculture, Volume 217, p. 108563. https://doi.org/10.1016/j.compag.2023.108563
