Factors Influencing Confidence in STEM Practices among Preservice Teachers
Corresponding email: teohsian@uitm.edu.my
Published at : 17 May 2024
Volume : IJtech
Vol 15, No 3 (2024)
DOI : https://doi.org/10.14716/ijtech.v15i3.6366
Teoh, S.H., Narayanan, G., Aris, S.R.S., Ibrahim, N., Isa, B., 2024. Factors Influencing Confidence in STEM Practices among Preservice Teachers. International Journal of Technology. Volume 15(3), pp. 687-696
| Sian Hoon Teoh | Department of Mathematics Education, Faculty of Education, Universiti Teknologi MARA, 42300 Bandar Puncak Alam, Selangor, Malaysia |
| Geethanjali Narayanan | Department of Teaching English as a Second Language, Faculty of Education, Universiti Teknologi MARA, 42300 Bandar Puncak Alam, Selangor, Malaysia |
| Sharipah Ruzaina Syed Aris | Department of Science Education, Faculty of Education, Universiti Teknologi MARA, 42300 Bandar Puncak Alam, Selangor, Malaysia |
| Norezan Ibrahim | Department of Science Education, Faculty of Education, Universiti Teknologi MARA, 42300 Bandar Puncak Alam, Selangor, Malaysia |
| Badrul Isa | Department of Art & Design Education, Faculty of Education, Universiti Teknologi MARA, 42300 Bandar Puncak Alam, Selangor, Malaysia |
Preservice teachers are
more confident in teaching mathematics, specifically when they have a better
understanding of the subject. In teaching and microteaching practices, content
and understanding of mathematics pedagogy are monitored and evaluated. However,
additional investigation on science, technology, engineering, and mathematics
(STEM) skills and practices is needed to meet the current need to train
teachers with 21st-century skills in order to educate future generations.
Therefore, this study aimed to determine the way in which factors influencing
effort, performances in algebra, and statistics were related to the
self-confidence of preservice teachers in teaching STEM practices. To achieve
this purpose, a correlational approach was used to collect information from 113
prospective science teachers at a public institution in Malaysia. In response
to the questionnaire, participants provided impressions of the experience and
level of confidence in using STEM. The result showed that the efforts of
preservice teachers had a significant impact on the confidence in using STEM
subjects in the mathematics classroom. Furthermore, the future of STEM
education was in the collective and practical efforts to connect STEM with the
real world.
Algebra; Confidence; Effort; Statistics; STEM (Science, Technology, Engineering and Mathematics)
Preservice teachers are evaluated based on
subject knowledge, engagement in teaching, and application of teaching theory (Asare and Amo, 2023; Stinken-Rösner et al., 2023; Cai,
Zhu, and Tian, 2022; Diamah et
al., 2022; Baier et al., 2021; Engin and Tasgin, 2021). Teachers
are expected to guide future generations toward academic and professional
excellence (VanDerHeide and
Marciano, 2022; Koh and Tan, 2021; Van-Driel and Berry, 2010), thereby
indicating the significance of STEM (science, technology, engineering, and
mathematics) skills in the current rapidly evolving digital world (Ryu, Mentzer and Knobloch,
2019).
With increasing attention being paid to STEM education, the integration of STEM
subjects into the real world by preservice teachers has become critical for
success (Syafril et al., 2021).
Learning and
teaching STEM subjects presents unique challenges for preservice teachers, as
they are in a transition period from practicing and supervising while preparing
to teach students (Pimthong and Williams, 2018). The
effectiveness of previous university studies in increasing the awareness of
integrating STEM elements into the curriculum remains a significant study gap.
To effectively
guide and inspire students, preservice teachers need to proactively develop
21st-century skills, particularly in STEM education. By connecting previous learning
experiences and current teaching practices, novice teachers can obtain valuable
insights to effectively guide the efforts. Additionally, teachers’
participation in STEM education assists in bridging the gap between theory and
practice and promotes the authenticity of STEM practices (Margot and Kettler, 2019;
Holmlund, Lesseig, and Slavit, 2018). This
implies that preservice teachers' willingness to put effort and actively engage
in STEM practices is critical to building self-confidence, strengthening
behavioral intentions, and creating more opportunities to engage students in
these activities (Teoh et al., 2022; Asvial, Mayangsari, and
Yudistriansyah, 2021; Margot and Kettler, 2019).
A strong
knowledge of algebra and statistics is essential for preservice mathematics
teachers to excel in STEM classes (Ng, 2019). This is
because a higher level of algebra knowledge helps in increasing confidence in
teaching mathematics concepts, and also allows teachers to effectively
introduce a broader range of STEM concepts. Therefore, preservice teachers who
have strong knowledge of algebra can confidently guide students in the
problem-solving process, such as when faced with a problem comprising the
concept of inverse proportionality in algebra. These problem-solving activities
are key to larger STEM activities, such as painting a house. By using algebra
knowledge, teachers can easily integrate additional STEM components into the
classroom by engaging students in discussions, such as a way of selecting
paints and brushes to paint efficiently and considering various factors that
influence painting speed.
Aside from the above, a strong foundation in algebra and statistics also
enables mathematics preservice teachers to excel in teaching concepts and
integrate a variety of STEM elements into the lessons, thereby providing
students with a more comprehensive and engaging educational experience.
However, these disciplines can be challenging and require more effort to
develop future students' talents (Khalil and Kier, 2021; Mergler and Spooner-Lane, 2012).
Assessing preservice teachers' confidence and effort in teaching STEM
practices, particularly algebra and statistics, provides an opportunity to
increase competency. The ability of teachers to effectively integrate the basic
mathematical concepts into real-world applications of STEM is critical to
preparing students for the demands of a rapidly evolving world of work that
relies heavily on STEM skills (Starr et al., 2022; Black et al., 2021; Ng,
2019). By focusing on the role of algebra and statistics in STEM education,
educational institutions and policymakers can develop targeted interventions to
strengthen preservice teachers' pedagogical skills and content knowledge in
these areas, thereby cultivating more competent and confident STEM educators.
This study aims to observe and understand the confidence level of
preservice mathematics teachers regarding their efforts and competence in
teaching STEM practices, with a particular focus on algebra and statistics.
Subsequently, this study provides answers for the following questions:
· First
Research Question: What are the levels of confidence, effort, and achievement
in algebra and statistics among preservice teachers?
· Second
Research Question: Is there a significant model that explains the relationship
between confidence in STEM practices and the independent variables of effort,
algebra, and statistics performance?
The results provide valuable insight into the
factors influencing the confidence of prospective teachers in STEM teaching
practices and help educational institutions and policymakers develop more
effective strategies to support and enable teachers to become competent. In
general, the goal is to improve the total quality of STEM education and create
a more dynamic and innovative learning environment for students.
2.1. Study Design
This study used a correlational design
to collect quantitative data. The quantitative approach focused on examining
the relationship between variables, namely confidence, effort, and mathematics
achievement (algebra and statistics).
2.2. Sampling
To collect quantitative data, this study used
cluster sampling, where samples were selected randomly from existing
categories. In this study, the cluster included the entire sample of 941
individuals who were divided into 37 clusters. The similarity between the
individuals in the population was that they enrolled in a preservice program in
the science and mathematics discipline at a Malaysian public university.
Additionally, the individuals had comparable characteristics that met the
selection criteria. These characteristics satisfied investigations, which
required the application of basic scientific knowledge, particularly algebra
and statistics. During the study period, this group of preservice teachers was
exposed to relevant information. The similarity of these characteristics was
important for cluster sampling because it allowed meaningful comparisons and
conclusions to be made regarding the particular group being studied (Raifman et al., 2022). Therefore,
the cluster sampling was used to gain a better understanding of preservice
teachers' experiences with STEM learning. Since all individuals in a given
cluster shared similar characteristics, data collected from each cluster would
possibly provide valuable insight into STEM learning experiences, attitudes,
and perceptions.
The sample consisted of 6 clusters totaling 37 clusters, which were selected randomly. Random sampling was a fundamental principle because it helped ensure that the sample was representative of the entire population and reduced the possibility of bias during the selection process. Consequently, the number of participants from the 6 clusters was 113 people, depending on the willingness to participate. The sample size was considered sufficient from a statistical perspective. According to the central limit theorem, sample sizes generally had to be large enough to provide statistical power and accurate estimates (Stroock, 2010). Additionally, the clusters were homogeneous to each other due to the science and mathematics-focused nature of the studies at the university, but the internally heterogeneous grouping was apparent as they were all selected to enrol in the program through an entrance selection process. To eliminate possible bias due to the relatively small number of clusters, bootstrap sampling was used in data analysis (Cameron, Gelbach, and Miller, 2008). Bootstrap sampling is a resampling method that comprised repeatedly taking random samples and replacing the samples from the original data set. This method allowed the estimation of sample variability and helped increase the robustness of statistical inference, particularly with limited cluster sizes.
2.3. Data Collection
The largest selection of preservice
teachers was in formation to hold a virtual meeting. Efforts were made to participate in the investigation
to ensure proper accountability. At the meeting, the overview of the
investigation was presented to preservice teachers and they responded to the
instrument.
2.4. Instrumentation
Participants responded to two
instruments, namely a questionnaire and a test. The primary instrument for
questions concerned the perceptions in the context of beliefs and efforts in
STEM practice. These building blocks were important aspects that needed to be
changed in the transformation of STEM education (Ministry of Education Malaysia, 2013).
Furthermore, the items were validated by two experts to establish valid
conditions. Also, the construct validity of the questionnaire was established
through factorial analysis. Based on the reliability test, Cronbach's alpha
values for effort and confidence were 0.896 and 0.899, respectively.
In the context of construct validity, KMO tests showed
adequate and high variability, with KMO = 0.889 > 0.50. This was because the
value was closer to 1, indicating high variability in the data. Additionally,
Bartlett test showed that there were sufficient correlations among the
variables with chi-square = 981.455, df = 66, and significance < 0.05. The result signified that the associated probability
was less than 0.05. Statistically,
there were two extracted factors which referred to components account for the
variance among the 12 variables. The percentage of variance accounted for by
the component was 67.85% of cumulative variance.
In this study, the two components were
rotated orthogonally, and the variable loadings (for some items) on the factors
were shown in Table 1. Subsequently, only factor loadings greater than 0.5 were
described. First, items 14, 15, 8, 6, and 7 were highly correlated to the first
component (confidence) with factor loadings of 0.873, 0.800, 0.786, 0.730, and
0.685, respectively. In this analysis, item 9 was rejected because the
variation in factor loadings between the two components was very small, namely
0.535 - 0.533 = 0.012, less than 0.2, which was called the limit value for
determining the remaining significant factor loadings. Second, the analysis
showed that items 1, 2, 3, 4, 12, and 13 for the second component (effort) were
highly correlated with factor loadings (for several items) of 0.873, 0.800,
0.786, 0.730, and 0.685, respectively.
Table 1 Rotated Component with a sample of the items
|
|
Component
1 |
Component
2 | |
|
14. My
confidence level in how to conduct STEM education is high. |
0.873 |
0.228 | |
|
15. I
am confident enough to introduce STEM education to my friends or community. |
0.800 |
0.384 | |
|
13. I
need to prepare myself to apply STEM in my practicum. |
0.384 |
0.629 | |
|
12. I
will put a lot of effort into supporting the development of STEM education. |
0.450 |
0.617 | |
The second instrument was an algebra and statistics test. In this process, the test questions were adapted from standardized examinations (California Department of Education, 2008a; 2008b). Several examples of the items were shown in Table 2. In the reliability test, Cronbach's alpha values for statistics and algebra were 0.795 and 0.620, respectively.
Table 2 Sample items
|
|
Sample item |
|
Statistics |
1. Rico’s first three test
scores in biology were 65, 90, and 73. What was his mean score? |
|
Algebra
|
1.
Lisa typed
a 1000-word essay at an average rate of 20 words per minute. If she started
typing at 6:20 p.m. and did not have any breaks, at what time did Lisa
finish typing the essay? 2.
Stephanie is reading a 456-page
book. During the past 7 days, she has read 168 pages. If she continues
reading at the same rate, how many more days will it take her to complete the
book? |
3.1.
Finding 1: Level of confidence in STEM practices
Finding
1 analyzed the data to provide descriptive statistics regarding
levels of trust and other factors. Subsequently, the following questions were
answered:
Question One: What are the levels of
confidence, effort, and achievement in algebra and statistics among preservice
teachers?
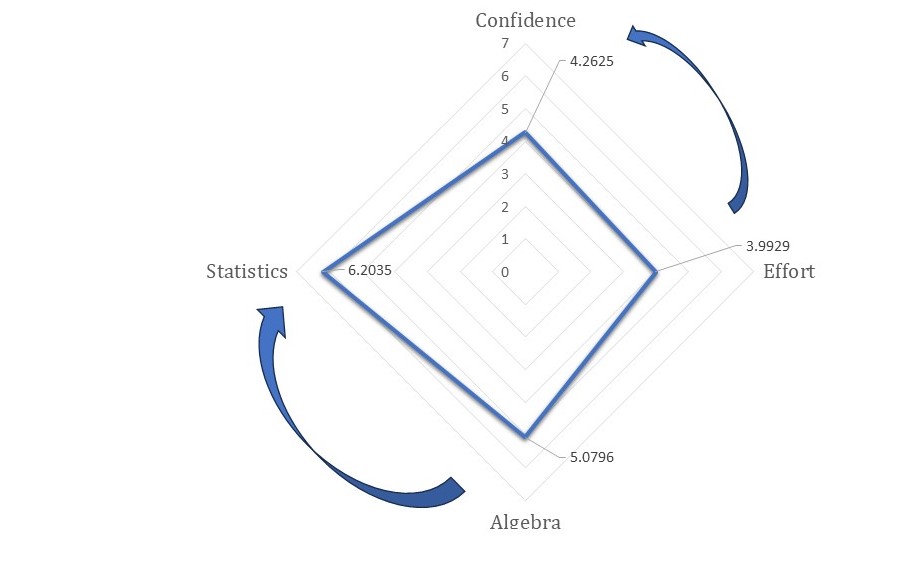
Confidence
and effort were measured on a Likert Scale ranging from ‘1’ for ‘strongly
disagree’ to ‘5’ for ‘strongly agree’. Table 3 shows the level of confidence
(mean = 4.263, standard deviation = 0.579) and effort
(mean = 3.993, standard deviation = 0.704). The result
implied that the level of confidence was rated slightly higher. Additionally,
it was observed that preservice teachers needed to put more effort into
studying algebra because their scores (in Table 3) were low, with mean = 5.080
(total score = 10 marks). The statistical performance was a bit higher (mean
=6.204) than algebra score.
Table 3 Descriptive Statistics
|
|
Statistic |
Bootstrapa
(1000 bootstrap samples) | ||||
|
Bias |
Std.
Error |
95%
Confidence Interval | ||||
|
Lower |
Upper | |||||
|
Confidence |
Mean, M |
4.263 |
-0.001 |
0.0555 |
4.153 |
4.366 |
|
Std. Deviation |
0.579 |
-0.006 |
0.0528 |
0.477 |
0.681 | |
|
N |
113 |
0 |
0 |
113 |
113 | |
|
Effort |
Mean |
3.993 |
-0.003 |
0.067 |
3.853 |
4.110 |
|
Std. Deviation |
0.704 |
-0.005 |
0.047 |
0.602 |
0.792 | |
|
N |
113 |
0 |
0 |
113 |
113 | |
|
Algebra Achievement (total =10
marks) |
Mean |
5.080 |
0.002 |
0.211 |
4.655 |
5.513 |
|
Std. Deviation |
2.311 |
-0.007 |
0.130 |
2.035 |
2.550 | |
|
N |
113 |
0 |
0 |
113 |
113 | |
|
Statistics Achievement |
Mean |
6.204 |
0.004 |
0.272 |
5.664 |
6.735 |
|
Std. Deviation |
2.860 |
-0.011 |
0.134 |
2.581 |
3.109 | |
|
N |
113 |
0 |
0 |
113 |
113 | |
3.2.
Finding 2: Model of confidence in
STEM practices
Finding
2 analyzed the data to present the inferential statistics of confidence level
and other factors. Subsequently, the following study question was answered:
Question Two: Is there a significant model
that explains the relationship between confidence in STEM practices and the
independent variables of effort, algebra, and statistics performance?
Least
square Regression with the stepwise method was conducted on the set of data,
which included dependent (confidence) and independent variables (effort,
algebra, and statistics performance). Table 4 shows the regression model with R2
= 0.546, indicating that 54.6% of the variation in the dependent variable
(confidence) was explained in the independent variable of effort but not by
other variables (algebra and statistics).
Table 4 Model Summary
|
Model |
R |
R
Square |
Adjusted
R Square |
Std.
Error of the Estimate |
|
1 |
0.739a |
0.546 |
0.542 |
0.392 |
|
a. Predictors: (Constant), Effort | ||||
The model was significantly presented since ANOVA analysis in
Table 5 shows that F = 133.346 with p < 0.05.
Table 5 ANOVAa
|
Model |
Sum
of Squares |
df |
Mean
Square |
F |
Sig. | |
|
1 |
Regression |
20.459 |
1 |
20.459 |
133.346 |
<0.001b |
|
Residual |
17.030 |
111 |
0.153 |
|
| |
|
Total |
37.489 |
112 |
|
|
| |
|
a.
Dependent Variable: Confidence; b. Predictors: (Constant), Effort | ||||||
According to Table 6, the model was significantly constructed
with only one independent variable (effort) for the dependent (Confidence).
Table 6 The Regression Model
|
Model |
Unstandardized
Coefficients |
Standardized
Coefficients |
t |
Sig. | ||
|
B |
Std.
Error |
Beta | ||||
|
1 |
(Constant) |
1.837 |
.213 |
|
8.613 |
<.001 |
|
Effort |
.608 |
.053 |
.739 |
11.548 |
<.001 | |
|
a.
Dependent Variable: Confidence | ||||||
According to Table 7, algebra and statistics performance did
not contribute to the model.
Table 7 Excluded Variables
|
Model |
Beta
In |
t |
Sig. |
Partial
Correlation |
Collinearity
Statistics | |
|
Tolerance | ||||||
|
1 |
Statistics Achievement |
0.028b |
0.433 |
0.666 |
0.041 |
0.977 |
|
Algebra Achievement |
0.060b |
0.915 |
0.362 |
0.087 |
0.964 | |
|
a.
Dependent Variable: Confidence;
b. Predictors in the Model: (Constant), Effort | ||||||
Although algebra and statistics performance did not contribute to the level of confidence, these two variables showed a fairly significant relationship. Table 8 shows that the correlation coefficient for the two variables was 0.646, where p < 0.05. The results also showed a strong positive relationship between confidence and effort, with the correlation coefficient being 0.739 (p < 0.05). Meanwhile, the relationship between effort and algebra performance was weak and negative, with a correlation coefficient of -0.19 (p < 0.05).
Table 8 Correlations
|
The
two Variables |
Pearson Correlation |
Sig. (2-tailed) |
95% Confidence Intervals
(2-tailed)a | |
|
Lower |
Upper | |||
|
Confidence
and Effort |
0.739 |
<0.001 |
0.640 |
0.811 |
|
Confidence
and Algebra Achievement |
-0.083 |
0.385 |
-0.263 |
0.104 |
|
Confidence
and Statistics Achievement |
-0.085 |
0.372 |
-0.265 |
0.102 |
|
Effort
and Algebra Achievement |
-0.190 |
0.044 |
-0.361 |
-0.004 |
|
Effort
and Statistics Achievement |
-0.152 |
0.108 |
-0.327 |
0.034 |
|
Algebra
Achievement and Statistics Achievement |
0.646 |
<0.001 |
0.521 |
0.741 |
4. Discussion
The results
suggested that preservice teachers could be more confident in STEM practices.
Influencing factors showed that teachers obtained confidence by completing
academic assignments and practicing independently. However, results in algebra
and statistics did not contribute to the degree of
certainty. Even though algebra and statistics were both important and related
to each other, as shown by the correlation coefficient, they did not contribute
to the level of confidence in STEM practices. The results showed a slightly
negative correlation between effort and algebra. This could be interpreted as
the need to try harder when teachers were weak in algebra. Therefore,
preservice teachers relied heavily on the efforts for any improvement. Despite
the results, teachers’ commitment to STEM practices significantly influenced
the development. It was suggested that universities needed to create more
assignments and projects to support STEM practices for preservice teachers.
Further
efforts were needed to develop pedagogy in
STEM teaching for preservice teachers, as it was suggested that teachers should
do more to develop the skills of the future students (Lee, Hsu, and Cheng, 2022; Khalil and
Kier, 2021; Leung, 2020; Pellas et al., 2020; Mergler and Spooner-Lane, 2012). However, equipping preservice
teachers with comprehensive and high-quality STEM knowledge, particularly
pedagogical knowledge, was a challenge. The challenges of teaching was in
informal educational experiences, such as integrating technological
tools into the learning environment (Gu et al., 2023; Guan et al., 2023; Love and Hughes, 2022; Neo et al., 2022). This scenario also showed the importance of
trying to acquire more experience in integrating STEM subjects into the
classroom. The experience could
come from informal activities, such as collaborative activities, which had been suggested to improve
preservice teachers' STEM skills (Berisha
and Vula, 2021; Kim and Keyhani, 2019).
Preservice
teachers also had lower algebra scores compared to the statistics. The results
suggested that preservice teachers needed training to improve algebra skills as
highlighted in previous studies (Johar et al.,
2023). Although there was a relationship between the two variables, namely
effort and algebra performance, the relationship was negative. This negative
sign indicated that more effort was needed for improvement.
In conclusion,
the implications of this study were categorized into three. First, it was
suggested that preservice teachers could acquire confidence in STEM practices
through their personal efforts, commitment, and practice. Therefore,
universities should develop more assignments and projects that explicitly
targeted STEM practices. This would enable preservice teachers to develop
greater confidence and competence in teaching STEM subjects. Second, this study
described the importance of developing pedagogical skills in STEM education for
prospective teachers. To teach STEM subjects effectively, preservice teachers
needed to have content and pedagogical knowledge that would enable teachers to
teach complex concepts effectively and engage students in meaningful learning
experiences. Several activitiess, such as integrating technology tools into the
learning environment and engaging in collaborative activities could also
contribute to preservice teachers' STEM knowledge. Third, this study described
the lower algebra scores of preservice teachers compared to the statistics, and
the negative correlation between effort and algebra performance. The results
suggested the need for targeted training and support to improve preservice
teachers' algebra knowledge. In summary, teachers could remain confident in
implementing STEM subjects by acquiring additional STEM-related knowledge in
order to have a strong foundation in both science and mathematics.
Additionally, the future of STEM education relied on collaborative and practical efforts
to connect STEM with real-world applications.
This
study is part of a larger study supported by the Special Research Grant (Geran
Penyelidikan Khas) from Universiti Teknologi MARA (UiTM). Grant:
600-RMC/GPK5/3 (040/2020).
| Filename | Description |
|---|---|
| R1-IE-6366-20230801172451.pdf | This is a list of corrections. |
Asare, P.Y., Amo, S.K., 2023. Developing Preservice Teachers’ Teaching
Engagement Efficacy: A Classroom Managerial Implication. Cogent Education,
Volume 10(1), pp. 1–17
Asvial,
M., Mayangsari, J., Yudistriansyah, A., 2021. Behavioral Intention of
e-Learning: A Case Study of Distance Learning at a Junior High School in
Indonesia due to the COVID-19 Pandemic. International Journal of Technology,
Volume 12(1), pp. 54–64
Baier, F., Maurer, C., Dignath, C., Kunter, M., 2021. Fostering
Pre-service Teachers’ Theoretical Knowledge Application: Studying with and
without Text-based Cases. Instructional Science, Volume 49(6), pp. 855–876
Berisha,
F., Vula, E., 2021. Developing Pre-service Teachers Conceptualization of STEM
and STEM Pedagogical Practices. Frontiers in Education, Volume 6, p.
585075
Black, S.E., Muller, C., Spitz-Oener, A., He, Z., Hung, K., Warren, J.R.,
2021. The Importance of STEM: High School Knowledge, Skills and Occupations in
an Era of Growing Inequality. Research Policy, Volume 50(7), p. 104249
Cai, Z., Zhu, J., Tian, S.,
2022. Preservice Teachers’ Teaching Internship
Affects Professional Identity: Self-efficacy and Learning Engagement as
Mediators. Frontiers in Psychology, Volume 13, p. 1070763
California
Department of Education, 2008a. Algebra and Functions. Available online at:
https://www.hemetadultschool.org/pdf/MATH_F.pdf, Accessed on October 8, 2022
California
Department of Education, 2008b. Data Analysis, and Probability. Available
online at:
http://ccasillas.weebly.com/uploads/3/0/8/0/30800917/stats,_probability.pdf,
Accessed on October 8, 2022
Cameron,
A.C., Gelbach, J., Miller, D.L., 2008. Bootstrap-Based Improvements for
Inference with Clustered Errors. The Review of Economics and Statistics,
Volume 90, pp. 414–427
Diamah, A., Rahmawati, Y., Paristiowati, M., Fitriani, E., Irwanto, I.,
Dobson, S., Sevilla, D., 2022. Evaluating the Effectiveness of Technological
Pedagogical Content Knowledge-based Training Program in Enhancing Pre-service
Teachers’ Perceptions of Technological Pedagogical Content Knowledge. Frontiers
in Education, Volume 7, p, 897447
Engin, M.Ç., Tasgin, A., 2021. Pre-service
Teachers’ Professional Commitment and their Ability to Choose Teaching
Techniques. Journal of Education and Future, Volume 21, pp. 29–40
Gu, X., Tong, D., Shi, P., Zou, Y., Yuan, H., Chen, C., Zhao, G., 2023.
Incorporating STEAM Activities into Creativity Training in Higher Education. Thinking
Skills and Creativity, Volume 50, p. 101395
Guan, J.-Q., Wang, L.-H., Chen, Q., Jin, K., Hwang, G.-J.,
2023. Effects of a Virtual Reality-based Pottery Making Approach on Junior High
School Students’ Creativity and Learning Engagement. Interactive Learning
Environments, Volume 31(4), pp. 2016–2032
Holmlund,
T.D., Lesseig, K., Slavit, D., 2018. Making Sense of “STEM Education” in K-12
Contexts. International Journal of STEM Education, Volume 5(1), pp. 1–18
Johar, R., Desy, D., Ramli, M., Sasalia, P., Walker, H.-C. O., 2023.
Preservice Teachers’ Noticing Skills in Relation to Student Misconceptions in
Algebra. European Journal of Educational Research, Volume 12(2), pp.
865–879
Khalil,
D., Kier, M., 2021. Equity-Centered Design Thinking in STEM Instructional
Leadership. Journal of Cases in Educational Leadership, Volume 24(1),
pp. 69–85
Kim, M. S., Keyhani, N., 2019. Understanding STEM Teacher Learning in an
Informal Setting: a Case Study of a Novice STEM Teacher. Research and
Practice in Technology Enhanced Learning, Volume 14(1), p. 9
Koh,
D., Tan, A.L., 2021. Singaporean Pre-service Teachers’ Perceptions of STEM
Epistemic Practices and Education. Journal of STEM Teacher Education,
Volume 56(2), pp. 1–30
Lee, S.W.-Y., Hsu, Y.-T., Cheng, K.-H., 2022. Do Curious Students Learn
More Science in an Immersive Virtual Reality Environment? Exploring the Impact
of Advance Organizers and Epistemic Curiosity. Computers & Education,
Volume 182, p. 104456
Leung, A., 2020. Boundary Crossing Pedagogy in STEM Education. International
Journal of STEM Education, Volume 7(1), p. 15
Love,
T.S., Hughes, A.J., 2022. Engineering Pedagogical Content Knowledge: Examining
Correlations with Formal and Informal Preparation Experiences. International
Journal of STEM Education, Volume 9(1), p. 29
Margot,
K.C., Kettler, T., 2019. Teachers’ Perception of STEM Integration and
Education: A Systematic Literature Review. International Journal of STEM
Education, Volume 6(1). pp. 1–16
Mergler,
A.G., Spooner-Lane, R., 2012. What Pre-Service Teachers Need to Know to Be Effective
at Values-Based Education. Australian Journal of Teacher Education,
Volume 37(8), pp. 66–81
Ministry
of Education Malaysia, 2013. Malaysia Education Blueprint 2013-2025 (Preschool
to PostSecondary Education). Putrajaya. Available online at: https://www.moe.gov.my/menumedia/media-cetak/penerbitan/dasar/1207
malaysia-education-blueprint-2013-2025/file, Accessed on October 8, 2022
Neo,
M., Lee, C.P., Tan, H.Y., Neo, T.K., Tan, Y.X., Mahendru, N., Ismat, Z., 2022.
Enhancing Students’ Online Learning Experiences with Artificial Intelligence
(AI): The MERLIN Project. International Journal of Technology, Volume
13(5), pp. 1023–1034
Ng,
S.B., 2019. Exploring STEM Competences for the 21st Century. UNESCO
International Bureau of Education. Available online at:
https://unesdoc.unesco.org/ark:/48223/pf0000368485, Accessed on October 8, 2022
Pellas, N., Dengel, A., Christopoulos, A., 2020. A Scoping Review of
Immersive Virtual Reality in STEM Education. IEEE Transactions on Learning
Technologies, Volume 13(4), pp. 748–761
Pimthong,
P., Williams, J., 2018. Preservice Teachers’ Understanding of STEM Education. Kasetsart
Journal of Social Sciences, Volume 2018, pp. 1–7
Raifman, S., DeVost, M.A.,
Digitale, J.C., Chen, Y.H., Morris, M.D. 2022. Respondent-Driven Sampling: A
Sampling Method for Hard-to-Reach Populations and Beyond. Current
Epidemiology Reports, Volume. 9 (1), pp. 38–47
Ryu, M., Mentzer, N., Knobloch,
N., 2019. Preservice Teachers’ Experiences of STEM Integration: Challenges and
Implications for Integrated STEM Teacher Preparation. International Journal
of Technology and Design Education, Volume 29(3), pp. 493–512
Starr, C.R., Ramos Carranza, P., Simpkins, S.D., 2022. Stability and
Changes in High School Students’ STEM Career Expectations: Variability Based on
STEM Support and Parent Education. Journal of Adolescence, Volume 94(6), pp.
906–919
Stinken-Rösner, L., Hofer, E., Rodenhauser, A., Abels, S., 2023.
Technology Implementation in Pre-Service Science Teacher Education Based on the
Transformative View of TPACK: Effects on Pre-Service Teachers’ TPACK,
Behavioral Orientations and Actions in Practice. Education Sciences, Volume 13(7), p. 732
Stroock,
D.W., 2010. Probability Theory: An Analytic View. 2nd Edition.
Cambridge University Press
Syafril,
S., Rahayu, T., Al-Munawwarah, S.F., Satar, I., Halim, L.B., Yaumas, N.E.,
Pahrudin, A., 2021. Mini Review: Improving Teachers’ Quality In STEM-Based
Science Teaching-Learning In Secondary School. Journal of Physics:
Conference Series, Volume 1796(1), p. 012072
Teoh, S.H., Mohamed, S.R., Mohd, A.H., Rasid, N.S.M.,
Yusof, M.M.M., 2022. Creating Engagement Opportunity for
Math Learning. International Journal of Technology, Volume 13(5), pp.
1013–1022
VanDerHeide,
J., Marciano, J.E., 2022. Preservice Teachers Taking Action: Enacting Teaching
Practices and Pedagogical Theories in an After-School Literacy Club Field
Experience. Journal of Teacher Education, Volume 73(2), pp. 175–187
Van-Driel,
J.H., Berry, A., 2010. Pedagogical Content Knowledge. In: International
Encyclopedia of Education. Third Edition, Elsevier, pp. 656–661
