Optoelectronic Characterization and Properties of Single-walled Carbon Nanotubes in a Liquid Dispersion Form
Corresponding email: sami_18001518@utp.edu.my
Published at : 18 Jan 2023
Volume : IJtech
Vol 14, No 1 (2023)
DOI : https://doi.org/10.14716/ijtech.v14i1.5124
Alabsi, S.S., Khir, M.H.M., Dennis, J.O., Hashwan, S.S.B., Algamili, A.S., 2023. Optoelectronic Characterization and Properties of Single-walled Carbon Nanotubes in a Liquid Dispersion Form. International Journal of Technology. Volume 14(1), pp. 65-77
| Sami S. Alabsi | Department of Electrical and Electronic Engineering, Universiti Teknologi PETRONAS, Seri Iskandar 32610, Malaysia |
| Mohd Haris Md. Khir | Department of Electrical and Electronic Engineering, Universiti Teknologi PETRONAS, Seri Iskandar 32610, Malaysia |
| John Ojur Dennis | Department of Fundamental and Applied Sciences, Universiti Teknologi PETRONAS, Seri Iskandar 32610, Malaysia |
| Saeed S. Ba Hashwan | Department of Electrical and Electronic Engineering, Universiti Teknologi PETRONAS, Seri Iskandar 32610, Malaysia |
| Abdullah S. Algamili | Department of Electrical and Electronic Engineering, Universiti Teknologi PETRONAS, Seri Iskandar 32610, Malaysia |
Carbon nanotubes (CNTs) are very promising
nanodevices due to their extraordinary electrical, thermal, and optical
properties. However, as-grown single-walled CNTs (SWCNTs) contain a mixture of
semiconducting and metallic species with great features versatility and
variation. Solution-based processing of this nanomaterial is vital for further
implementation in applicable platforms. In this paper, important optoelectronic
intrinsic properties of SWCNTs in dispersions are studied applying a
semi-empirical approach of optical characterization and Tauc/Davis-Mott
relation and Max-Planck equations. SWCNTs are found to have a direct bandgap of
2.20 eV, an indirect bandgap of 0.27 eV, an optical conductivity of about 107
S cm-1, and exhibited a metamaterial behavior ascribed to the high
negative permittivity. In addition, the optimum parameters for the dispersion
of SWCNTs, and the separation of the semiconducting species using simple
mechanical methods of ultrasonication and density gradient ultracentrifugation,
respectively, without using surfactants are also presented.
Carbon nanotubes; Dispersion; Optoelectronic properties; Separation; Spectroscopy
CNTs
are allotropes of the carbon family with hollow cylinder structures. Based on
the chirality angle divergence of the graphene sheet, their electronic
properties may vary between metallic and semiconducting CNTs exist as
single-walled, dual-walled or multiwalled (Setyopratomo et al., 2018; Thanthirige et al., 2016). Consisting only of surface (i.e., no bulk C-atoms), they are extremely
sensitive and responsive to surface modification and optical excitation. This
peculiarity of 1D ultra-thin structure renders CNTs as a potential material for
future high-performance scaled nanotechnology (Alabsi et al., 2020; Sudibandriyo et
al., 2015).
The optoelectronic properties of SWCNTs are important features and determinants
of many applications of these nanodevices.
Optoelectronic
applications of SWCNTs are increasingly gaining more attention due to the
extreme sensitivity and responsivity of these nanodevices to light energy.
Absorption of light observes the momentum and energy conservation laws which imply that the photo-energy sufficient for the
excitation of electrons from ground Anomalous optoelectronic behavior of SWCNTs
may, however, be associated with structural defects and/or surface
functionalization.
Thus,
spectroscopy of the intrinsic properties of pristine SWCNTs is fathomable by
analysis in the range of UV-Vis and NIR spectra. In fact, optical
characterization is the preferred technique and is perhaps the simplest method
of studying the intrinsic properties of dispersion based SWCNTs (Eremina et al., 2017; Cheung et al.,
2016; Nguyen and Shim, 2015;). It paves the way for their implementation in
many optoelectronic applications (Mousavi, 2012). It is also a tool to
investigate the phases of several processes in real time, such as the quality
of the dispersion and separation of metallic and semiconducting nanotubes (Yang et al., 2016). Optical analysis may
reveal numerous optoelectronic properties of SWCNTs such as optical
transmittance (Tsapenko et al., 2018), conductivity and dielectric
properties (Smirnov et al., 2019;
Smirnov et al., 2018), energy bandgap, and optical absorption
coefficient (El-Moussawi et al., 2019; Saad et al., 2019; Blancon et al.,
2015).
Intriguingly, SWCNTs exhibit extraordinary
but anisotropic properties. This anisotropicity stems from the nanotubes
variation in several factors such as diameter, chirality, and length (Sharma and Jaggi,
2017; Ba Hashwan et al., 2015).
Despite numerous studies on the optical and electronic characteristics of
SWCNTs, knowledge of their optoelectronic conductivity, bandgap energy, and
dielectric behavior is still a matter of ongoing research. For instance,
Mousavi reported that larger SWCNT diameter is associated with lower bandgaps
and hence higher optical conductivity (Mousavi,
2012). Kuwahara, Hirai, and Saito
(2018), on the other hand, demonstrated that a thin
film SWCNTs network of smaller diameter tubes showed better conductivity
performance. Length of the nanotube may also be a factor determining the
optoelectronic features of the SWCNTs. Shorter tubes are associated with less
light transmittance and lower conductivity (Farbod,
Zilaie, Kazeminezhad, 2017).
He et al. (2016)
reported an extremely anisotropic conductivities of perpendicularly (~42 Scm-1)
and parallelly (~2500 Scm-1) aligned SWCNTs electronic devices. The
bandgap of the nitrophenyl functionalized SWCNTs was calculated between 1.19 eV
and 2.60 eV by (El-Moussawi et al., 2019).
Kawale et al. (2016)
obtained an average bandgap of 1.23 eV for samples synthesized at 700? C, while
reporting a nearly 0 eV indirect bandgap for all carbon films. On the contrary,
a large window of a direct bandgap of 6 eV and indirect bandgap of 5.2 eV of
pristine CNTs was reported by (Murali and Perumal,
2018). Nonetheless, the absorption spectra have
shown some variation over several studies. In one study, the UV-Vis absorption
peaks of SWCNTs solution were reported at 225 nm and 273 nm (Attal, Thiruvengadathan, and Regev, 2006),
while absorption maxima were observed at ~206 nm and ~251 nm by another study (Rance et al., 2010).
?-plasmon absorption with two extreme peaks at ~246 and ~275 nm were recorded
by (Cheung et al., 2016).
2.1. Materials
The materials used in this study were of analytical grade. Pristine
unfunctionalized SWCNTs in powder form produced by arc-discharge technique was
obtained from Iljin Nanotech. The polar solvents of dimethyl formamide (DMF)
and tetrahydrofuran (THF), and DI water (18.2 M? cm) were purchased from
Sigma-Aldrich. All materials were used as received. Experiments were conducted
at the nanomaterials laboratory, Universiti Teknologi Petronas.
2.2. Methodology
2.2.1. Dispersion and separation
Solution based SWCNTs was prepared by
adding the powder form of pristine as-synthesized SWCNTs
to DMF in three different concentrations: 0.1%, 0.01%, and 0.001% ?/?. The
mixture was then ultrasonicated, using Jeken ultrasonicator PS-40A, at four
different sonication durations: 30min, 60min, 90min, and 120min, at a moderate
power of 40W. The use of surfactants was avoided to preserve the intrinsic
properties of the nanotubes.
For the separation of the semiconduction
(sc)-CNTs from the metallic (m)-CNTs, 0.01% w/v aqueous solution of SWCNTs in
DI water was stirred at 300 rpm for 30 min at room temperature, then
ultracentrifuged at 15,000 rpm ~ 27720 RCF, using Benchtop High Speed
Refrigerated Centrifuge BCBHR-308, at four different cycle and duration
settings: 30 min, 60 min, 90 min, 120 min. The supernatant yield was carefully
fractioned for optical analysis after each centrifugation cycle. The algorithm
is related to the density gradience between the two electronic species with the
semiconducting being buoyant with less dense gradient extracted from the top
phase of the yield. In other words, ultracentrifugation at this gravity force
might be effective in the separation of nanotubes suggesting gradient of
metallic nanotubes is denser than semiconducting ones.
2.2.2. Characterization
Spectroscopy of absorbance/reflectance
of light in the ultraviolet, visible and near infrared regions was performed
using UV-Vis NIR spectroscopy (Agilant, Carry 60) with a dual beam mode, a scan
rate of 4800 nm/min, starting wavelength of 1100 nm and a stopping wavelength
of 190 nm. Correction/subtraction of the blank spectra (i.e., DMF solvent) from
the sample by dual-beam spectrometer was reckoned for to obtain the absorbance
due purely to the CNT species.
3.1. Absorption Spectra and Dispersion
The transition that occurs from the ground state to the
excited state requires the absorption of a specific amount of energy given by E = hv, where h is the
Planck’s constant and v is the
frequency of the incident light.
The energy difference between HOMO and LUMO corresponds to
the electron excitations pertaining to absorption of a specific light
wavelength spectrum. The broad range of wavelengths in Figure 1 is, in fact,
explained by the CNT 1D structure density of states known as Van Hove
singularities. The transition that takes place at the wavelength that absorbs
most strongly at 275 nm for 0.1% concentration, and at 280 nm for both 0.01%
and 0.001% concentrations represent the ?-plasmon absorbance.
Figure 1 Absorbance spectra relative to variation of
sonication durations against variation of concentration: (A) 0.1%, (B) 0.01%,
and (C) 0.001% of SWCNT/DMF.
The increase in sonication time had a significant effect on increasing the absorbance intensity in all concentrations. At the highest concentration of 0.1%, the increase of absorbance intensity over sonication durations was somehow monotonic. Lower concentrations had higher rate of SWCNTs deagglomeration evident by the increment in the absorbance intensities. Dramatic increase, however, takes place at 120 min/0.01%, 90 min/0.001% and even more sizable leap is observable at 120 min/0.001% reaching as much as double the absorbance intensity as that of 120 min/0.1%. Higher absorbance intensities signify higher rate of debundling and homogeneity of the dispersions (Nguyen et al., 2011). The absorbance is remarkably showing two significant peaks: small and major. The major absorbance peak of 0.1% occurs at 275 nm but a shift towards the red zone is observed as concentration decreases with peaks center at 280 nm for 0.01% and 0.001%. The small peak at 236 nm remains unshifted regardless of the change in concentration and sonication duration. The major peaks represent the strong ?-plasmon resonance absorbance peaks of the nanotubes in liquid form (Smirnov et al., 2019). Similar strong ?-plasmon peaks were observed by (Li et al., 2009). Table 1 demonstrates the prominent peaks along with their respective wavelengths for all concentrations and sonication durations.
Table 1 Absorbance intensities and corresponding wavelengths of major peaks for each duration setting and concentration
Monotonic decrease in absorbance intensity after the major peak of 0.1% and 0.01% is observed without showing further significant isolated peaks. Note that only after 120min/0.001% the major peak is now contorted to include two more peaks. The inclusion of the two peaks of 310 nm and 370 nm, along with the significant increase in the absorbance intensities, especially at 120 min, is an indication of a deeper and more significant deagglomeration of the SWCNTs and confirms that the dispersion has improved over sonication durations.
Figure 2 Deconvolution of the
120 min/0.001% setting peak
The
interband excitonic transitions are more evident and wider, which implies
higher rate of deagglomeration and the existence of individually dispersed
SWCNTs at higher rates in the lower concentration dispersions, specifically at
the highest sonication duration of 120 min than in the higher concentrations
and at lower sonication durations. The deconvolution analysis of the highest
peak of the 120 min/0.001% setting in Figure 2 illustrates the breakdown of the
individual nanotube transitions. It reveals the mixture composition and the
variation of the nanotubes’ properties between metallic and semiconducting as
the absorption shifts from the UV to the visible and near infrared regions. The
increment in the absorbance intensities is also an indication of better dispersibility
and debundling of individual nanotubes over extended sonication durations (Jiang, Song, and Xu, 2018). Moreover, the few
peaks along the wavelength spectrum is an indication of the purity of the
nanotube species
and the low rate of contamination.
However,
DMF is a neutral Lewis base that readily facilitates the catalysis of
the reversible process of deprotonation of the nanotubes (Arai, 2010). The shift in the
peak waveform towards the NIR zone may also be an indication of the
deprotonation of the carbon nanotubes with longer sonication durations (Kozlowska, Meyer, and Rodziewicz, 2019; Nguyen and Shim, 2015). It can
also be ascribed to the swelling, ionization, and dissociation of the nanotube
species in the DMF. While metallic SWCNTs are more reactive to protonation near
neutral pH, semiconducting SWCNTs are reactive and sensitive to protonation
near more acidic pH values (Engtrakul et al., 2005). Protonation is a
reversible process whereby SWCNTs neither lose their electron, nor do they
change structure of electronic state permanently.
Absorbance spectra presented here, are
in accordance with the results obtained by (El-Moussawi et al., 2019). Similar
waveforms were also observed in other works ( Nguyen and Shim, 2015; Nguyen et al., 2011).
In comparison, other studies reported
longer sonication durations, and some resorted to the use of surfactants which
are difficult to remove and known to degrade the performance of the nanotubes. Mougel et al. (2016) reported
the successful dispersion of SWCNTs at an optimum sonication time of 2.5 h
using a power density of 0.7 W/mL. Another study showed that SWCNTs retained
most of their length after 2 h sonication of the nanotubes in SDBS at 90 W (Gomulya et al., 2013). At a
higher power of 225 W, Barman et al. (2010)
investigated the optical characteristics of SWCNTs in NMP at a range of
different sonication durations reaching up to 480 min. The absorption intensity
increased and peaked at 120 min but notably decreased after this duration point
due to re-bundling that was confirmed by AFM images. While in a different
study, a tip-sonication was performed to disperse SWCNTs in sodium deoxycholate
(DOC) for 1.5 h in an ice water bath at a power of 1 W/mL. Raman analysis of
the nanotubes lengths indicated a low level of defects (Simien et al., 2008).
3.2. Separation of sc-SWCNTs
The optical absorption bands of the sc-SWCNTs buoyant density fraction is illustrated in Figure 3. Isolated nanotubes absorb light at different wavelengths allowing for the determination of their conductivity type. The photoresponse of the metallic (M11) electronic transition is observed in the visible region, while the photoresponse of the semiconducting (S22) electronic transition of the nanotubes is observed in the near infrared region (Ong, Euler, and Levitsky, 2010). The complete attenuation of the absorption spectra at the M11 transition band of the metallic nanotubes and the notable increase in the absorption spectra at the S22 transition band of semiconducting nanotubes is an indication of the successful separation of the sc-CNTs from m-CNTs. Notably, the absorption peaks of the metallic nanotubes transition bands faded away with longer DGU durations, while absorption peaks at the semiconducting absorption band gained stronger pulse at higher DGU durations. Fractions after each ultracentrifugation setting contained higher purity yield of semiconducting nanotubes. This is evident by the inclusion of more peaks with higher intensities at the S22 excitonic band and the demise of the peaks at the M11 excitonic band due to the consistent increasing rate of gradient buoyancy density differentiation of the sc-CNTs and the m-CNTs with increased ultracentrifugation duration settings. Absorbance peaks in the S22 excitonic band are attributed to the Van Hove singularities absorption in the NIR region. Results here are in agreement with results by (Wang and Lei, 2020; Tang et al., 2018; Eremina et al., 2017).
Figure 3 Metallic and
semiconducting electronic transitions
The separation of the
sc-SWCNTs from the m-SWCNTs was further validated by the extrapolation of the
bandgap energies discussed in following section.
3.3. Bandgap
The bandgap of a material is the difference of energy that an electron
is required to have in order to make a transition from one band of lower energy
to another of higher energy. Based on the energy required to make this
transition, the absorption of photo-energy in the UV-Vis NIR spectroscopy is
correspondent to specific molecular orbitals (MO). As aforementioned, these
orbitals represent bonding and antibonding orbitals, and the transition takes
place between the HOMO and the LUMO. The absorption at the red edge of the
spectra is utilized to determine the bandgap. The bandgap is obtained using
equation:
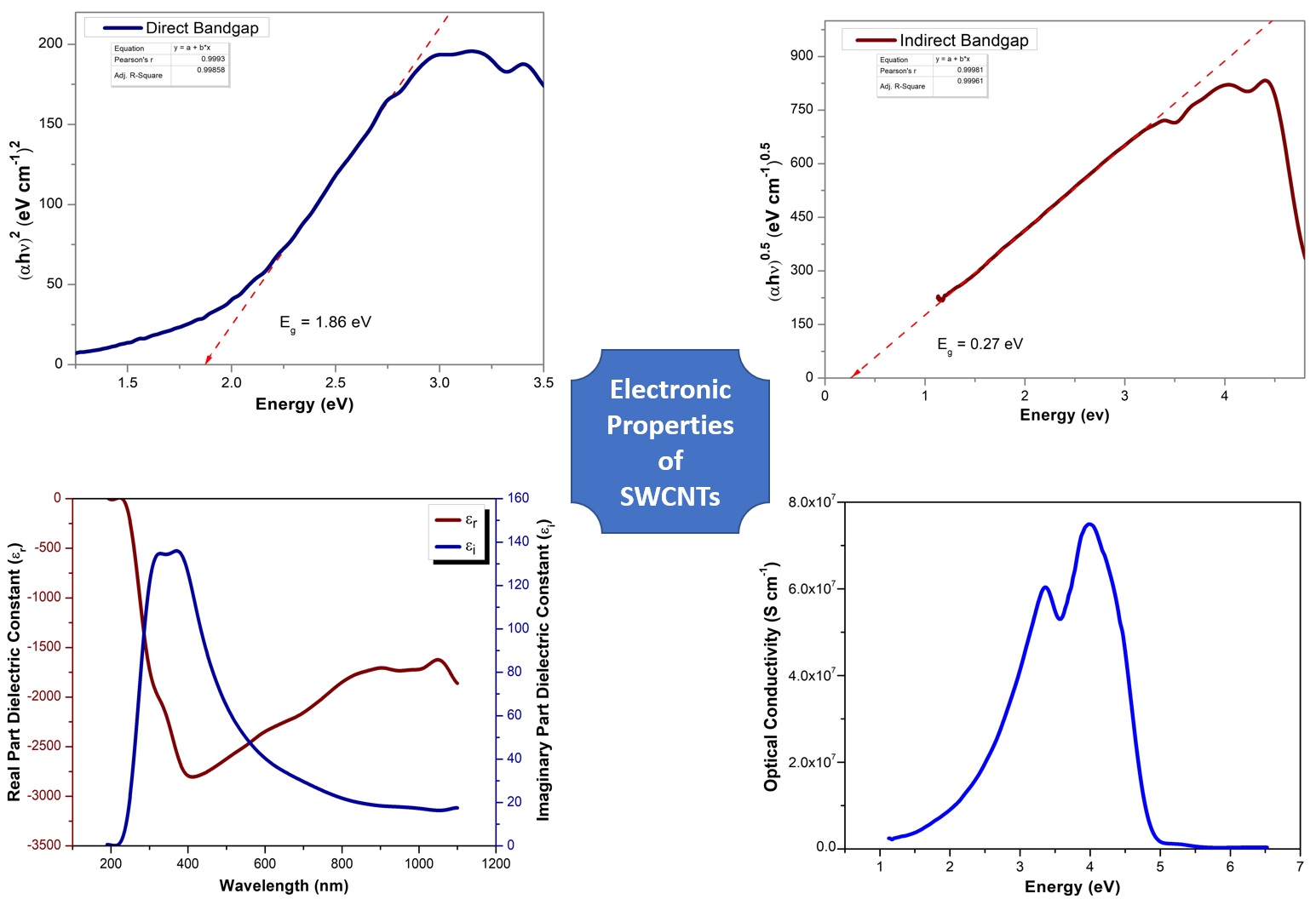
where ? is the absorbance coefficient, and the collective term (hv) is denoting the incident photon energy. The exponent “n” represents the nature of transition. For direct transition n = 2 and for indirect transition n = 1/2. K is energy independent constant, and Eg is the optical bandgap energy.
Figure 4 Tauc-Davis Mott
direct and indirect band gap transitions for (A) before and (B) after DGU
The extrapolation of
the bandgaps in Figure 4 demonstrates that SWCNTs are direct and indirect
nanomaterial. There is a significant disparity between the direct bandgap and
the indirect one obtained from the samples before DGU. This is ascribed to the
nature of the as-synthesized nanotubes having a mixture of both metallic and
semiconducting species.
The indirect bandgap at 0.27 eV is showing a quasi-metallic behavior of
the nanotubes, while the 2.20 eV direct bandgap is more to the semiconducting
nano-species, both existing in the as-synthesized mixture. The bandgap is
inversely proportional to the nanotube diameter. A 2.20 eV bandgap might be
associated to a CNT with a diameter of a 0.2 nm, while a small bandgap could be
of a CNT with a larger diameter (Venkataraman et al., 2019).
However, after the DGU, the direct and indirect bandgap disparity has
significantly shrunken, and the difference was reduced to a lower range. In
Figure 4, the values of 1.86 eV and 2.28 eV, for the direct and indirect
bandgaps, respectively, are both in the semiconducting range, which is believed
to be due to the separation of the metallic nanotubes from the semiconducting
ones.
Indirect bandgap of CNTs was reported by one study (Wang et al., 2017). SWCNTs
may change, under strain, from direct to indirect gaps as explained by (Qiu et al., 2016). While,
another study construed that the nanotubes are of direct bandgap due to their
1D structure (Li et al., 2009). Murali and Perumal (2018) extrapolated the bandgap of SWCNT at 6 eV
and 5.2 eV for direct and indirect transitions, respectively, using x-intercept
in Tauc plot.
According to a probable direct inter-band transitions between the Van
Hove singularities and the density of states, three optical bandgaps of 2.2 eV,
3.5 eV, and 4 eV were obtained by (Gharbavi and Badehian, 2015). In another study
based on density functional methods, it was showed that armchair (n, n) SWCNTs
have indirect semiconducting bandgap ranging 1.062 eV - 2.112 eV (n = 3 – 10),
while for zigzag (n, 0), the SWCNTs have a direct semiconducting bandgap
ranging 0.659 eV - 1.603 eV (n = 5 – 10) (Sun, 2011).
3.3. Optical Conductivity
Optical conductivity is another
important parameter in the optoelectronic analysis of CNTs. It unlocks the
potential for numerous applications of CNTs in optoelectronic field (Mousavi, 2012). The
optical conductivity was mathematically modelled and graphed against
the excitation wavelengths and against the incident photon energy.
Optical conductivity depends mainly on the
optical bandgap, refractive index, absorption coefficient, incident photon
frequency and excitation coefficient and is given by the following equation (Arbab, 2019; Sharma and Katyal, 2007):
where ![]() is the refractive index, and
is the refractive index, and ![]() is the speed of light in vacuum. The
refractive index is given by:
is the speed of light in vacuum. The
refractive index is given by:
where is the percentage of transmittance, and is the light absorbance, which is a
dimensionless quantity.
|
|
|
Figure 5 Optical conductivity
of SWCNTs as a function of (A) wavelength and (B) optical energy
Figure 5
depicts the optical conductivity of the SWCNTs. The most prominent peak centers
at ~3.9 eV, similar to results reported by (Gharbavi and Badehian, 2015). Another
smaller peak at ~3.35 eV is also evident. This means that SWCNTs are most
conducting at optical energy bands of ~3.35 - 3.9 eV. Moreover, it is observed
that the maxima of electron excitation are attained at wavelengths 310 nm and
370 nm. SWCNTs have a high conductivity in the order of 107 - 108
as also reported by (Venkataraman et al., 2019; Zhang et al., 2015). While, a
non-resonant optical conductivity of individual SWCNTs was measured at by another study (Blancon et al., 2015).
3.4. Dielectric
The
dielectric behavior of SWCNTs is given by are the dielectric constant real and imaginary
parts, respectively. n is the refractive index. K is the extinction coefficient, and
is the absorption coefficient obtained by the
following equations:
Figure 6 The real and the
imaginary parts of SWCNTs dielectric behavior
The
permittivity properties of the nanotubes are tunable to light wavelengths and
intensities (Smirnov et al., 2019). The
dielectric behavior of SWCNTs can be examined against wavelength, frequency or
energy (Sharma and Jaggi, 2017). Figure
6 is demonstrating the dielectric complex of the real and imaginary parts. The
permittivity of SWCNTs, given by the real part, decreases drastically below
zero to high negative values in the UV range but increase at visible and near infrared
wavelengths to lower negative values. While the real part decreases, the
imaginary part increases in the UV region, and they start to reverse direction
beyond the UV wavelength range towards the visible and NIR regions. The
imaginary part indicates that there is a large loss of energy or absorption of
light whereby electrons start to be excited to conduction levels. However, the
loss of the imaginary part never tends to zero as is the case of pure
dielectric materials, while the permittivity of the real part, though
increased, remains negative. This behavior Indicates that SWCNTs demonstrate a
ferroelectric and metamaterial properties (Flory, 2011). High
negative values of the real part of dielectric in the range of -11000 to -7000
is also observed by Zhang and colleagues. According to Zhang et al., radius and
electrical conductivity of the CNT bundles are the main contributors of the
real part permittivity negative value (Zhang et al., 2015). The
change in conductivity is related to excitation of surface plasmons depending
on the illumination of light wavelength in the visible and NIR regime (Smirnov et al., 2019). Li and
Liu also obtained a negative real part permittivity of the SWCNT samples and
explained that the increase and decrease in the real and imaginary parts of the
SWCNTs dielectric constant follow the Drude model of free electron movement (Li and Lue, 2007). Similar results by
Smirnov et al. showed that with higher augmented light intensities, the
real part can, however, increase to positive values (Smirnov et al., 2018). This
study pointed out that the real part exhibits resonant behavior that the
conductivity cannot be explained by the simple Drude model but rather by using
Drude-Lorentz model combined with the Maxwell-Garnett theory.
Optical
characterization with semi-empirical extrapolation using Tauc/Davis and
Max-Planck models can be a powerful tool to reveal significant optical and
electronic properties of SWCNTs. The high conductivity and the versatile dielectric
behavior are features claiming SWCNTs as the nanomaterial for a futuristic wide
range of applications. In addition, analysis of the absorption spectra revealed
the successful dispersion and separation of SWCNTs that was achieved using an
organic solvent along with simple mechanical processing methods, without
incorporating any additives. The parameters of sonication and
ultracentrifugation durations and concentration were studied. Higher durations
of sonication at lower concentrations yielded the optimum homogeneous
dispersion, while the separation of semiconducting nanotubes was validated
against absorption intensities and bandgap energies.
This research was
supported by the Universiti Teknologi PETRONAS, Malaysia, through the Graduate
Assistantship (GA) Scheme.
Alabsi, S.S., Ahmed, A.Y., Dennis, J.O.,
Khir, M.H.M., Algamili, A.S., 2020. A review of carbon nanotubes field
effect-based biosensors. IEEE Access, Volume 8, pp. 69509–69521
Arai, T., 2010. 8.2.16.1 Lewis base catalysis. Science
of Synthesis Knowledge, Volume 4, p. 224
Arbab, A.I., 2019. On the optical
conductivity. Optik, Volume 194, p. 163067
Attal, S., Thiruvengadathan, R., Regev, O.,
2006. Determination of the concentration of single-walled carbon nanotubes in
aqueous dispersions using UV-visible absorption spectroscopy. Analytical
Chemistry, Volume 78(23), pp. 8098–8104
Ba Hashwan, S.S., Fatin, M.F., Ruslinda,
A.R., Md Arshad, M.K., Hashim, U., Ayub, R.M., 2015. Functionalization of multi
wall carbon nanotubes using nitric acid oxidation. Applied Mechanics and
Materials, Volume 754–755, pp. 1156–1160
Barman, S.N., Lemieux, M.C., Baek, J., Rivera,
R., Bao, Z., 2010. Effects of dispersion conditions of single-walled carbon
nanotubes on the electrical characteristics of thin film network transistors. ACS
Applied Materials and Interfaces, Volume 2(9), pp. 2672–2678
Berwal, N., Dhankhar, S., Sharma, P., Kundu,
R.S., Punia, R., Kishore, N., 2017. Physical, structural and optical
characterization of silicate modified bismuth-borate-tellurite glasses. Journal
of Molecular Structure, Volume 1127, pp. 636–644
Blancon, J., 2015. Optical absorption and
electronic properties of individual carbon nanotubes To cite this version?: e
par carbone individuels. Master’s Thesis,
Graduate Program, Université Claude Bernard Lyon 1, France
Bouazza, A., Bassaid, S., Daho, B., Messori,
M., Dehbi, A., 2021. Synthesis and characterization of a composite organic
semiconductor (curcumin-paracetamol/TiO2). Polymers and Polymer Composites,
Volume 29(5), pp. 417–426
Cheung, W., Patel, M., Ma, Y., Chen, Y., Xie,
Q., Lockard, J.V., Gao, Y., He, H., 2016. ?-Plasmon absorption of carbon
nanotubes for the selective and sensitive detection of Fe3+ ions. Chemical
Science, Volume 7(8), pp. 5192–5199
Chiodarelli, N., De Volder, M., 2019.
High-throughput and consistent production of aqueous suspensions of Single-Wall
Carbon Nanotubes. Carbon, Volume 145, pp. 757–763
El-Moussawi, Z., Nourdine, A., Medlej, H.,
Hamieh, T., Chenevier, P., Flandin, L., 2019. Fine tuning of optoelectronic
properties of single-walled carbon nanotubes from conductors to semiconductors.
Carbon, Volume 153, pp. 337–346
Engtrakul, C., Davis, M.F., Gennett, T.,
Dillon, A.C., Jones, K.M., Heben, M.J., 2005. Protonation of carbon
single-walled nanotubes studied using13C and1H-13C cross polarization nuclear
magnetic resonance and Raman spectroscopies. Journal of the American
Chemical Society, Volume 127(49), pp. 17548–17555
Eremina, V.A., Obraztsov, P.A., Fedotov,
P.V., Chernov, A.I., Obraztsova, E.D., 2017. Separation and optical
identification of semiconducting and metallic single-walled carbon nanotubes. Physica
Status Solidi (B) Basic Research, Volume 254(5), p. 1600659
Farbod, M., Zilaie, A., Kazeminezhad, I.,
2017. Advanced materials and devices carbon nanotubes length optimization for
preparation of improved transparent and conducting thin fi lm substrates. Journal
of Science: Advanced Materials and Devices, Volume 2(1), pp. 99–104
Flory, F., 2011. Optical properties of
nanostructured materials: a review. Journal of Nanophotonics,
Volume 5(1), p. 052502
Gharbavi, K., Badehian, H., 2015. Optical
properties of armchair (7,7) single walled carbon nanotubes. AIP Advances,
Volume 5(7), p. 077155
Gomulya, W., Costanzo, G.D., De Carvalho,
E.J.F., Bisri, S.Z., Derenskyi, V., Fritsch, M., Fröhlich, N., Allard, S.,
Gordiichuk, P., Herrmann, A., Marrink, S.J., Dos Santos, M.C., Scherf, U., Loi,
M.A., 2013. Semiconducting
single-walled carbon nanotubes on demand by polymer wrapping. Advanced
Materials, Volume 25(21), pp. 2948–2956
He, X., Gao, W., Li, B., Zhang, Q., 2016. Wafer-scale
monodomain films of spontaneously aligned single-walled carbon nanotubes. Nature Nanotechnology, Volume 11, pp. 633–638
Jiang, Y., Song, H., Xu, R., 2018. Research
on the dispersion of carbon nanotubes by ultrasonic oscillation, surfactant and
centrifugation respectively and fiscal policies for its industrial development.
Ultrasonics Sonochemistry, Volume 48, pp. 30–38
Johan, M.R., Suhaimy, S.H.M., Yusof, Y., 2014. Physico-chemical studies
of cuprous oxide (Cu 2 O) nanoparticles coated on amorphous carbon nanotubes
(?-CNTs). Applied Surface Science, Volume 289, pp. 450–454
Kawale, S.S., Afre, R., Sharon, M., Bhosale,
C.H., Sharon, M., 2016. Carbon nanotube?: An indirect ~ 0 eV band gap
material Volume 11(7), pp. 3546–3550
Kim, S.L., Choi, K., Tazebay, A., Yu, C.,
2014. Flexible power fabrics made of carbon nanotubes for harvesting
thermoelectricity. ACS Nano, Volume 8(3), pp. 2377–2386
Kozlowska, M., Meyer, B., Rodziewicz, P.,
2019. Single-walled carbon nanotubes in tetrahydrofuran solution:
microsolvation from first-principles calculations. Journal of Molecular
Modeling, Volume 25(7), p. 206
Kuwahara, Y., Hirai, T., Saito, T., 2018.
Effects of tube diameter and length on transparent conductivity of
single-walled carbon nanotube network films. Journal of Nanomaterials,
Volume 2018, p. 5393290
Li, X., Zhu, H., Wei, J., Wang, K., Xu, E.,
Li, Z., Wu, D., 2009. Determination of band gaps of self-assembled carbon
nanotube films using Tauc/Davis-Mott model. Applied Physics A: Materials
Science and Processing, Volume 97(2), pp. 341–344
Li, Y.H., and Lue, J.T., 2007. Dielectric constants of single-wall carbon nanotubes at
various frequencies. Journal of Nanoscience and Nanotechnology, Volume
7(9), pp. 3185–3188
Mougel, J., Adda, C., Bertoncini, P., Capron,
I., Cathala, B., Chauvet, O., 2016. Highly e ffi cient and predictable noncovalent dispersion of single- walled
and multi-walled carbon nanotubes by cellulose nanocrystals. Journal of Physical Chemistry C, Volume 120(39), pp.
22694–22701
Mousavi, H., 2012. Optical conductivity of
carbon nanotubes. Optics Communications, Volume 285(13–14), pp.
3137–3139
Murali, S., Perumal, S., 2018. Synthesis of
nanotubes under carbon environment at low temperature using hydrothermal
method. Optik, Volume 162, pp. 81–85
Nguyen, T.T., Nguyen, S.U., Phuong, D.T., Nguyen, D.C., Mai, A.T.,
2011. Dispersion of denatured carbon nanotubes by using a dimethylformamide
solution. Advances in Natural Sciences: Nanoscience and Nanotechnology,
Volume 2(3), p. 035015
Nguyen, V. H., Shim, J. J., 2015. Green
synthesis and characterization of carbon nanotubes/polyaniline nanocomposites. Journal
of Spectroscopy, Volume 2015, p. 297804
Njuguna, J., Vanli, O.A., Liang, R., 2015. A review
of spectral methods for dispersion characterization of carbon nanotubes in
aqueous suspensions. Journal of Spectroscopy, Volume 2015, p. 463156
Ong, P.L., Euler, W.B., Levitsky, I. A.,
2010. Hybrid solar cells based on single-walled carbon nanotubes/Si
heterojunctions. Nanotechnology, Volume 21(10), p. 105203
Qiu, M., Xie, Y., Gao, X., Li, J., Deng, Y., Guan, D., Ma, L.,
Yuan, C., 2016. Band gap opening and semiconductor-metal phase transition in
(n, n) single-walled carbon nanotubes with distinctive boron-nitrogen line defect.
Physical Chemistry Chemical Physics, Volume 18(6), pp. 4643–4651
Rance, G.A., Marsh, D.H., Nicholas, R.J.,
Khlobystov, A.N., 2010. UV-vis absorption spectroscopy of carbon nanotubes:
Relationship between the ?-electron plasmon and nanotube diameter. Chemical
Physics Letters, Volume 493(1–3), pp. 19–23
Saad, I. Ben, Hannachi, N., Roisnel, T.,
Hlel, F., 2019. Optical, UV-Vis spectroscopy studies, electrical and dielectric
properties of transition metal-based of the novel organic-inorganic hybrid (C6
H10 N2)(Hg2Cl5)2.3H2O. Journal of Advanced Dielectrics, Volume 9(5), pp.
1–15
Setyopratomo, P., Wulan, P.P.D.K.,
Sudibandriyo, M., 2018. The effect of metal loading on the performance of
tri-metallic supported catalyst for carbon nanotubes synthesis from liquefied
petroleum gas. International Journal of Technology, Volume 9(1), pp.
120–129
Sharma, D., Jaggi, N., 2017. Static
refractive index engineering of a singlewalled carbon nanotube through
co-doping: A theoretical study. Optik, Volume 131, pp. 267–272
Sharma, P., Katyal, S. C., 2007.
Determination of optical parameters of a-(As2Se 3)90Ge10 thin film. Journal
of Physics D: Applied Physics, Volume 40(7), pp. 2115–2120
Simien, D., Fagan, J.A., Luo, W., Douglas,
J.F., Migler, K., Obrzut, J., 2008. Influence of nanotube length on the optical
and conductivity properties of thin single-wall carbon nanotube networks. ACS
Nano, Volume 2(9), pp. 1879–1884
Smirnov, S., Anoshkin, I.V., Demchenko, P.,
Gomon, D., Lioubtchenko, D.V., Khodzitsky, M., Oberhammer, J., 2018. Optically controlled dielectric
properties of single-walled carbon nanotubes for terahertz wave applications. Nanoscale,
Volume 10(26), pp. 12291–12296
Smirnov, S., Anoshkin, I.V., Generalov, A.,
Lioubtchenko, D.V., Oberhammer, J., 2019. Wavelength-dependent
photoconductivity of single-walled carbon nanotube layers. RSC Advances,
Volume 9(26), pp. 14677–14682
Sudibandriyo, M., Wulan, P.P.D.K., Prasodjo,
P., 2015. Adsorption capacity and its dynamic behavior of the hydrogen storage
on carbon nanotubes. International Journal of Technology, Volume 6(7),
pp. 1128–1136
Sun, J., 2011. Stability and electronic
structures of single-walled AlP nanotubes by first principle study. Procedia
Engineering, Volume 15, pp. 5062–5066
Tan, K.H., Ahmad, R., Leo, B.F., Yew, M.C.,
Ang, B.C., Johan, M.R., 2012. Physico-chemical studies of amorphous carbon
nanotubes synthesized at low temperature. Materials Research Bulletin,
Volume 47(8), pp. 1849–1854
Tang, J., Cao, Q., Tulevski, G., Jenkins,
K.A., Nela, L., Farmer, D.B., Han, S.J., 2018. Flexible CMOS integrated
circuits based on carbon nanotubes with sub-10 ns stage delays. Nature
Electronics, Volume 1(3), pp. 191–196
Tan, W.H., Lee, S.L., Ng, J., Chong,
W.W.F., Chong, C.T., 2016. Characterization of carbon
nanotubes synthesized from hydrocarbon-rich flame. International Journal of
Technology, Volume 7(2), pp.
343–351
Tsapenko, A.P., Goldt, A.E., Shulga, E., Popov, Z.I., Maslakov, K.I.,
Anisimov, A.S., Sorokin, P. B., Nasibulin, A.G., 2018. Highly conductive and
transparent films of HAuCl4-doped single-walled carbon nanotubes for flexible
applications. Carbon, Volume 130, pp. 448–457
Venkataraman, A., Amadi, E.V., Chen, Y.,
Papadopoulos, C., 2019. Carbon nanotube assembly and integration for
applications. Nanoscale Research Letters, Volume 14(1), p. 220
Wang, J., Lei, T., 2020. Separation of
semiconducting carbon nanotubes using conjugated polymer wrapping. Polymers,
Volume 12(7), pp. 1–29
Wang, W., Xu, J., Zhang, Y., Li, G., 2017.
First-Principles Study of Electronic Structure and Optical Properties of
Silicon/Carbon Nanotube. Computational Chemistry, Volume 05(04), pp.
159–171
Yang, M-c., Li, M-y, Luo,
S., Liang, R., 2016. Real-time monitoring of carbon nanotube dispersion using
dynamic light scattering and UV-vis spectroscopy. International Journal of
Advanced Manufacturing Technology, Volume 82(1–4), pp. 361–367
Zhang, W., Xiong, H., Wang, S.,
Li, M., Gu, Y., 2015. Negative permittivity behavior of aligned carbon nanotube
films. Applied Physics Letters, Volume 106(18), pp. 3–8
